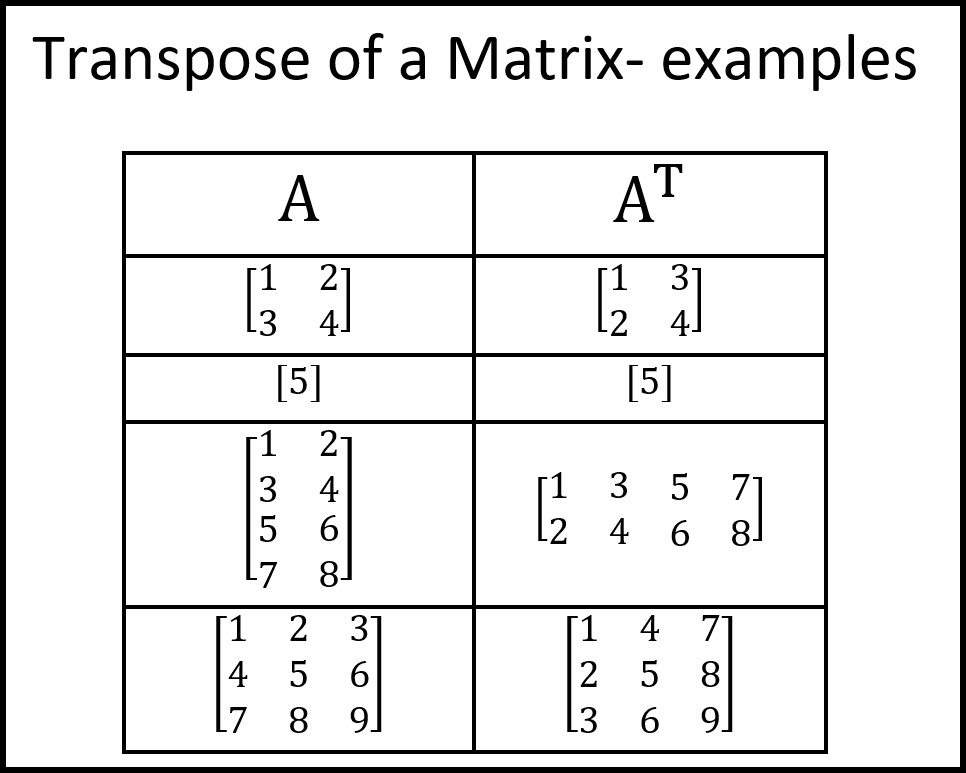
The transpose of a matrix is created by interchanging its rows and columns. The transpose of a matrix are denoted as \(A’\) or \(A^{\text{T}}\).
Notes

Questions & Solutions
\(\textbf{1)}\) Take the Transpose of this Matrix
\( \left[ {\begin{array}{ccc}21 & 22 & 23 \\41 & 42 & 43 \\81 & 82 & 83 \end{array} } \right]\)

\(\textbf{2)}\) Take the Transpose of this Matrix
\( \left[{\begin{array}{ccc} 1 \\ 2 \\ 5 \end{array} } \right]\)
\(\textbf{3)}\) Take the Transpose of this Matrix
\( \left[{\begin{array}{ccc} 6 & 7 \\ 3 & 8 \\ 9 & 0 \end{array} } \right]\)
\(\textbf{4)}\) Take the Transpose of this Matrix
\( \left[{\begin{array}{cc} 1 & 2 \\ 3 & 4 \end{array} } \right]\)
\(\textbf{5)}\) Take the Transpose of this Matrix
\( \left[{\begin{array}{c} 7 & -8 & 9 \end{array} } \right]\)
\(\textbf{6)}\) Take the Transpose of this Matrix
\( \left[{\begin{array}{c} 4 \end{array} } \right]\)
See Related Pages\(\)
\(\bullet\text{ Matrix Transpose Calculator (Symbolab.com)}\)
\(\bullet\text{ Intro to Matrices}\)
\(\,\,\,\,\,\,\,\,\)\(\left[ {\begin{array}{ccc}4 & -5 & 2 \\1 & 0 & 3 \\\end{array} } \right]\)
\(\bullet\text{ Matrix Operations}\)
\(\,\,\,\,\,\,\,\,\)\( \left[ {\begin{array}{ccc}3 & 45 & 6 \\-8 & 2 & 4 \\1 & 0 & 3 \\\end{array} } \right]\)\(+\left[ {\begin{array}{ccc}3 & 45 & 6 \\-8 & 2 & 4 \\1 & 0 & 3 \\\end{array} } \right]\)
\(\bullet\text{ Multiplying Matrices}\)
\(\,\,\,\,\,\,\,\,\)\(\left[{\begin{array}{cc} 1 & 2 \\ -3 & -4 \\\end{array} } \right]\)\(\left[ {\begin{array}{cc}6 & -3 \\5 & 0 \\\end{array} } \right]\)
\(\bullet\text{ Determinants}\)
\(\,\,\,\,\,\,\,\,\)\(\left|{\begin{array}{cc} a & b \\ c & d \\ \end{array} } \right|=ad-bc\)
\(\bullet\text{ Cramer’s Rule}\)
\(\,\,\,\,\,\,\,\,\text{ax+by=e } \& \text{ cx+dy=f}…\)
\(\bullet\text{ Identity Matrix}\)
\(\,\,\,\,\,\,\,\,\)\(\left[{\begin{array}{ccc} 1 & 0 & 0\\ 0 & 1 & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]\)
\(\bullet\text{ Identity and Inverse Matrices}\)
\(\,\,\,\,\,\,\,\,A^{-1}=\displaystyle\frac{1}{ad-bc}\left[{\begin{array}{cc} a & b \\ c & d \\ \end{array} } \right]\)
\(\bullet\text{ Transpose Matrix}\)
\(\,\,\,\,\,\,\,\,\left[{\begin{array}{ccc} 1 \\ 2 \\ 5 \\ \end{array} } \right]\Rightarrow\left[{\begin{array}{c} 1 & 2 & 5 \end{array} } \right]\)
\(\bullet\text{ Rotation Matrix}\)
\(\,\,\,\,\,\,\,\,\)\(R(\theta)=\left[{\begin{array}{cc}\cos{\theta} & -\sin{\theta} \\\sin{\theta} & \cos{\theta} \\\end{array} } \right]\)
\(\bullet\text{ Eigenvectors and Eigenvalues}\)
\(\,\,\,\,\,\,\,\,(A-\lambda I)\vec{v}=\vec{0}\)
\(\bullet\text{ Andymath Homepage}\)
About Andymath.com
Andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. If you have any requests for additional content, please contact Andy at [email protected]. He will promptly add the content.
Topics cover Elementary Math, Middle School, Algebra, Geometry, Algebra 2/Pre-calculus/Trig, Calculus and Probability/Statistics. In the future, I hope to add Physics and Linear Algebra content.
Visit me on Youtube, Tiktok, Instagram and Facebook. Andymath content has a unique approach to presenting mathematics. The clear explanations, strong visuals mixed with dry humor regularly get millions of views. We are open to collaborations of all types, please contact Andy at [email protected] for all enquiries. To offer financial support, visit my Patreon page. Let’s help students understand the math way of thinking!
Thank you for visiting. How exciting!
