Volume of Hollow Cylinder

In Geometry, we have learned about different solid shapes such as cylinders, cubes, cuboids, cones and so on. The hollow cylinder is also a three-dimensional shape. The cylinder that is empty from the inside and has some thickness at the peripheral is known as a hollow cylinder. In this article, we are going to learn the volume of hollow cylinder, its formula, derivation and examples in detail.

Volume of Hollow Cylinder Formula

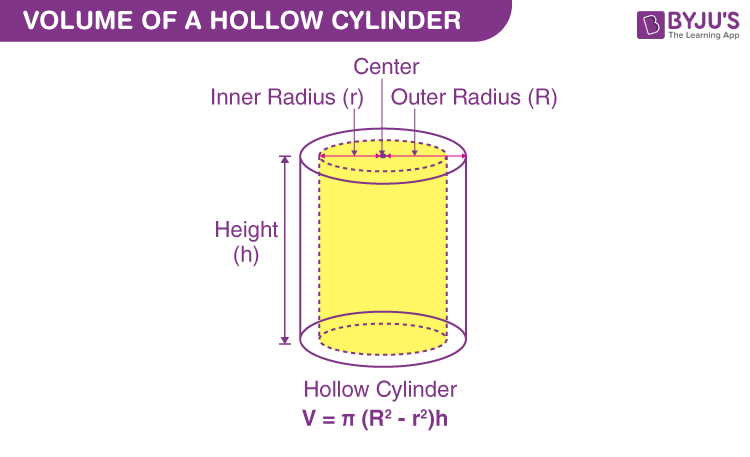
The hollow cylinder is defined as a cylinder, which is empty from inside and has some difference between the internal and external radius. The bottom of the hollow cylinder looks like an annular ring. In other words, the bottom of the hollow cylinder resembles a region bounded by two concentric circles.

If both the outer and inner radius of a hollow cylinder along with the height is given, then the volume of the hollow cylinder is given by the formula:

Where,

“R” is the outer radius, “r” is the inner radius, and “h” is the height of the hollow cylinder.

Volume of Hollow Cylinder Derivation

We know that the volume of cylinder is Base area × Height = (πr²) × h cubic units

Where “r” is the radius and “h” is the height of the cylinder.

Thus, the volume of a hollow cylinder can be calculated by subtracting the volume of the internal cylinder from the volume of the external cylinder.

(i.e) Volume of Hollow cylinder with outer radius “R” and inner radius “r” and height “h” = Volume of a cylinder with radius “R” and height “h” – Volume of a cylinder with radius “r” and height “h”.

⇒The volume of hollow cylinder = πR²× h – πr²× h

⇒ V = π (R² -r²)h cubic units

Hence, the volume of the hollow cylinder, V = π (R² -r²)h cubic units, is derived.

Steps to Find the Volume of a Hollow Cylinder

The following are the steps to find the volume of the hollow cylinder:

Step 1: Identify the given dimensions of the hollow cylinder, such as inner radius “r”, outer radius “R” and height “h” and make sure that all have the same units.

Step 2: Substitute the given values in the volume of hollow cylinder formula V = π (R² -r²)h.

Step 3: Finally, represent the answer along with the unit.

Volume of Hollow Cylinder Examples

Example 1:

Find the volume of a hollow cylinder whose outer radius is 7 cm and inner radius is 5 cm and height is 7 cm. (Use π = 22/7)

Solution:

Given:

Outer Radius = 7 cm

Inner Radius = 5 cm

Height = 7 cm.

To find: Volume “V”.

We know that the formula for the volume of a hollow cylinder is π (R² -r²)h cubic units.

Substituting the known values in the formula, we get

V = (22/7)(7² – 5²)7 cm³

V = 22(49-25) cm³

V = 22(24) cm³

V = 528 cm³

Therefore, the volume of a hollow cylinder’s outer radius is 7 cm, inner radius is 5 cm and height is 7 cm is 528 cm³.

Example 2:

Find the height of the hollow cylinder given that volume = 314.15 cm³, outer radius = 6 cm and inner radius = 4 cm. (Use π =3.14)

Solution:

Given:

Outer radius, R = 6 cm

Inner Radius, r = 4 cm

Volume = 314.15 cm³

To find: Height “h”

As we know, the volume of hollow cylinder formula is, V = π (R² -r²)h cubic units

Now, substitute the known values

314.15 = (3.14)(6²-4²)h

314.15/3.14 = (36-16)h [314.15/3.14=100.047, which is rounded to 100]

100 = 20h

h= 100/20

h= 5

Hence, the height of the hollow cylinder is 5 cm.

Example 3:

Determine the inner radius of a hollow cylinder whose outer radius is 5 cm, height is 10 cm and volume is 502.4 cm³. (Use π =3.14)

Solution:

Given: Outer radius, R = 5 cm

Height = 10 cm

Volume = 502.4 cm³

To find: Inner radius “r”.

Substituting the values in the volume of the hollow cylinder formula,

V = π (R² -r²)h cubic units

502. 4 = (3.14) (5² – r²)(10)

(502.4)/(31.4) = 5² – r²

16 = 5² – r²

16 = 25 – r²

r² = 25-16

r² = 9

r = 3.

Therefore, the inner radius of the hollow cylinder r is 3 cm.

Practice Question

Solve the following problems:

1. Find the volume of a hollow cylinder whose outer radius is 3 m, inner radius is 1 m and height is 4m. (Use π =3.14).
2. Find the outer radius of the hollow cylinder whose inner radius is 2 cm, height is 8 cm and volume is 527.78 cm³.
3. Find the height of the hollow cylinder whose volume is 829.38 cm³, outer radius is 12 cm, and inner radius is 10 cm.