The Platonic solids are 3-dimensional shapes that nature uses to build itself. The mental construct of reality, seen in the form of geometry. There are only five, which resonates with the (phi)ve aspect of life.
Let’s imagine you are on the inside of a sphere and are asked to make a bunch of different three-dimensional forms using only straight lines that all touch the surface of the sphere. You could make all kinds, an infinite amount actually. But if every line had to be of equal length, and all the shapes made had to be the same, you could only make 5 different ‘perfect solids’ if you will.
These have been coined the ‘Platonic solids’, for his namesake, Plato. Before his time they called them the Pythagorean solids because Pythagoras taught them in his mystery school. There is no original ‘inventor’ of these solids because they were not an invention. They were discovered and it is debatable who first took notice. Stone balls carved as these solids have been found in England and Scotland, dating back 5000 years. They were probably known of thousands of years before prior. As Manly P. Hall once wrote: “Mother Nature has shaken many civilizations from her back.”
These perfect solids are an intrinsic quality of our geometric reality. There is esoteric knowledge hidden in the way Nature structures itself, the most obvious being that there are five of these forms. There can be only five. You can prove this to yourself conceptually.
To make 3-D forms, first we need to start out with 2-D shapes. Two points on a piece of paper can’t make a shape, but three can, so the first shape is the triangle, the second is the square, and the third is the pentagon. The fourth shape is the hexagon but if you try to put a bunch of hexagons together to make a 3-D form it just doesn’t work. They leave gaps in-between. Only triangles, squares, and pentagons can be combined three dimensionally to create ‘Platonic solids’. Let’s just call them ‘perfect solids’ because it’s debatable who first discovered them.
We can connect three equilateral triangles together to make a point. One more is required to fill in the other side for a total of four triangles. This is called the tetra-hedron, the first perfect solid. Tetra means four, and ‘hedron’ means sided. Plato associated this solid with Fire because its pointy ends mimic the stabbing sensation of a flame. Spicy food burns your mouth because of its tetrahedral composition at its molecular level. At this point you’re sticking out your tongue trying to get more air, which leads us to our next solid, the Octahedron.
Four triangles fit together to make a pyramid. Picture the great one at the Giza plateau. However a pyramid is not a Platonic solid, since there’s a different shape at its base, a square. If we took two great pyramids and put them together at their square base we would create the second most complex perfect solid; the octahedron, which symbolizes Air according the Plato and many other sources.
‘Octa’ means eight. But, don’t let Octo-ber fool you being the tenth month of the year; Pope Gregory messed up the calendar 1500 years ago (I think..) The first month is actually March, which makes a lot more sense. In this regard, Sept-ember, Oct-ober, Nov-ember, and Dec-ember all fall on their respective etymologically numeric moon-ths. Sept means 7, Octo means 8, Nov means 9, and Dec means 10. Maybe we should correct Pope Greg’s egregious mistake and fix the calendar.
Now, back to geometry. If we took five equilateral triangles and put them together at one point this would begin the construction of an icosahedron. Icosa, meaning twenty. We would have to add 16 more triangles to complete this solid. This was associated with Water perhaps due to its ball-like shape, similar to a bead of water. The icosahedron is the least stagnant and most spherical of the five solids. If we took six triangles and put them together we would see that they form a flat surface, so this is no good. We can’t make any more of these perfect solids with triangles alone. Logically we have to go on to the next shape to make more perfect solids. Would you like to join us in this geometric adventure!? Be there, or be…… square.
The cube is the only perfect solid made of squares. Three squares join together to make a point. If we put four squares together at one point, we’d just get another larger square which is not three-dimensional.
A cube is also called a hexa-hedron. Hexa means six. Hedron means sided. So we have a six-sided perfect solid made of squares. Plato associated the cube and the square with Earth. The sphere or circle would symbolize Heaven or the “One Mind”.
Square has etymological roots in ‘quad’, which means four. There is something about four that is fundamental, more so than three, even. You would think three would come first in the process of creation. To satisfy this thought-experiment, draw a circle on graph paper using a compass. Expand the radius with the point of the compass on one of the graph cross-sections. Within the circle, drawing a square is a lot easier than trying to draw a triangle. The question is: Did the Creator use graph paper? Probably not.
The dodecahedron is the last of the perfect solids and is composed of pentagons. Dodecahedron means twelve sided. The five sides consist of pentagons which reflect the total number of perfect solids and encode the golden number which is inherent in all life. Three penta-gons (five-sided shapes) fit together at one point. Nine are added to complete the construction of the most mysterious and ineffable of all the perfect solids.
There are 360° degrees in a circle, 180° degrees in a triangle, 360° degrees in a square, and 540° degrees in a pentagon. With this information we can figure out the total angles in each of the solids. This information will prove to be useful when we are looking at distances in the cosmos, measured in ancient imperial units, like miles, furlongs, and feet.
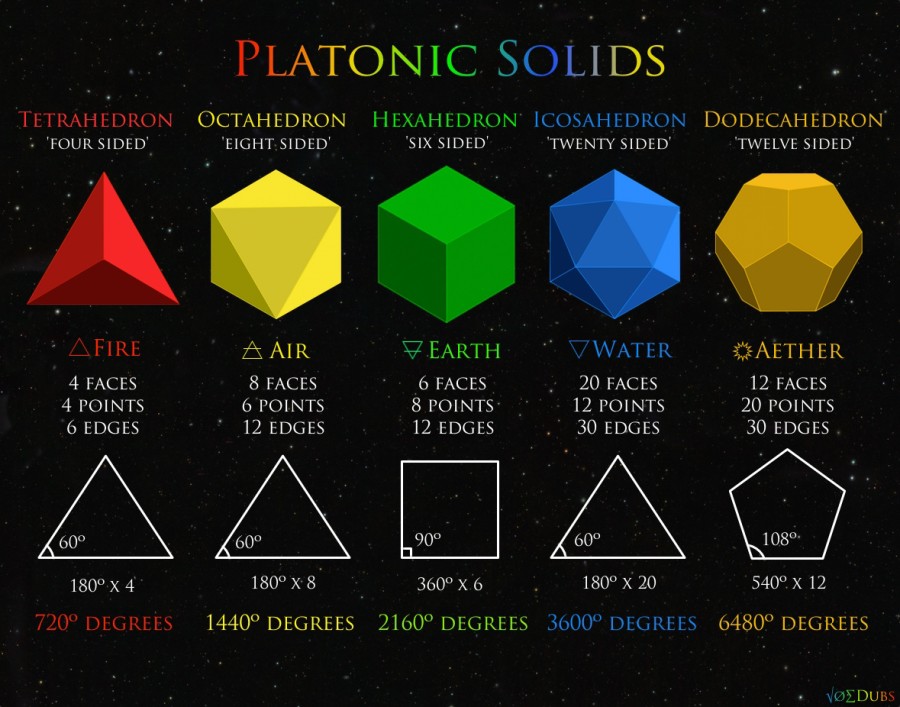
The five ‘perfect solids’ total 14,400 degrees total in their angular measure. 144 is a very auspicious number, highly revered by the Ancients
The 3-D geometry of the universe is based on these perfect solids. This knowledge was not invented, but discovered. It’s inherent in all things. This geometry is the armature or structure of the matrix that makes up our reality.
3,4, and 5 are the first numbers that combine to form the first so called ‘Pythagorean Triple’, which obeys the rule ‘A squared plus B squared equals C squared’ (See…high school geometry did come in handy after all). The physical sizes of Earth and Moon encode the 3-4-5 triangle, which itself also encodes Phi, and therefore encodes life.
Like our five senses there are five Platonic solids, each of which is made up of shapes that have 3,4, or 5 sides. The tetrahedron is made up of 4 triangles(3-sided), the octahedron, 8 triangles. And the icosahedron has 20 triangles. The cube is composed of 6 squares(4-sided) and the dodecahedron is made of 12 pentagons(5-sided). Three-Four-Phive is reflected in the way nature structures itself, based upon the simplest 3-D geometries in our reality.

Can you see the three-four-fiveness? Both in the shapes and how the shapes meet? Perhaps we should call them Pythagorean solids since they encode his famous 3-4-5 triangle?
In addition the Platonic solids also encode 3-4-5 in the way the shapes fit together. The tetrahedron, cube, and dodecahedron have three shapes at their corners. The octahedron, four. And the icosahedron has five shapes coming together at every point. Again, the Three-Four-Phiveness, in the way Nature structures itself.
“The harmony of the world is made manifest in Form and Number, and the heart and soul and all the poetry of Natural Philosophy are embodied in the concept of mathematical beauty.” -D’Arcy Wentworth Thompson
“Geometry is knowledge that appears to be produced by human beings, yet whose meaning is totally independent of them.” -Rudolf Steiner
“Pythagoras was one of the first teachers to establish a community wherein all the members were of mutual assistance to one another in the common attainment of the higher sciences. He also introduced the discipline of retrospection as essential to the development of the spiritual mind. Pythagoreanism may be summarized as a system of metaphysical speculation concerning the relationships between numbers and the causal agencies of existence.” -Manly P. Hall
- Tetrahedron – Fire
- Octahedron – Air
- Hexahedron – Earth
- Icosahedron – Water
- Dodecahedron – Aether
- Platonic Solids
artist: I am an Astronaut song: Winter Solstice album: Aether Experiment

















Hi Jo. Great art. The infographics really help simplify everything. Are you familiar with the Moon Model of the atomic nucleus?
http://www.21stcenturysciencetech.com/articles/moon_nuc.html
http://www.21stcenturysciencetech.com/articles/moonart2.html#Figure 1
Hey Swamiananta, I have heard of Robert Moon and his platonic solid model of the elements. I forgot all about that. Thanks for reminding me I might have to add this info the the post 🙂 . Great links thanks for sharing!
So, how do we know there are only 5 since there are infinite numbers? How high has anyone gone to prove this?
explained above, which part don[t you innerstand broski.
The part where it’s explained. I don’t see anything beyond 5. Has anyone proven that no other polygon with n sides can become a.perfect 3d object within a sphere? I don’t see where this has been addressed.
Try cutting out shapes like triangles squares, pentagons, and maybe hexagons?
Put them together and try to make a 3-d shape that :
1. has all identical shapes
2. all points of the shape touch the outside of a sphere (pretend a ball is around it.
You can only make 5, try it.
https://www.mathsisfun.com/geometry/platonic-solids-why-five.html
http://mathonline.wikidot.com/proof-of-the-existence-of-only-5-platonic-solids
Yes, I understand this part. What I was asking is how many sides has this been tried for? For instance, has it ever been documented where a person has attempted this with a 37 sided object or a 105 sided object? What is the upper limit at which it was decided these are the only 5 shapes that work?
I just ask out of personal curiosity. These are the kind of questions which plague my life 🙂
“Clearly it’s impossible for four (or more) pentagonal faces to meet at a vertex, because they subtend more than 360 degrees. For hexagonal (six-sided) faces, three hexagons meeting at a point constitute another “infinite solid”, i.e., a planar surface. It’s also obvious that no higher-order polygon can yield a solid, so the five solids already mentioned – tetrahedron, hexahedron, octahedron, icosahedron, and dodecahedron – are the only regular polyhedrons.”
https://www.mathpages.com/home/kmath096/kmath096.htm
However, if you bend the rules:
http://theconversation.com/after-400-years-mathematicians-find-a-new-class-of-solid-shapes-23217
These are solid shapes that do not conform to the rules of the platonic solids, but can still be useful.
To see examples: Google “new platonic solid”
Technical math details:
http://mathworld.wolfram.com/PlatonicSolid.html