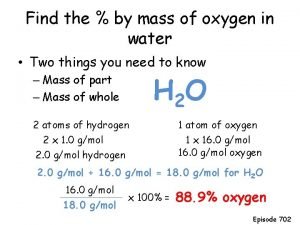Water Quality Management in Rivers Dissolved Oxygen Depletion























- Slides: 36

Water Quality Management in Rivers

Dissolved Oxygen Depletion

Biochemical Oxygen Demand Measurement • Take sample of waste; dilute with oxygen saturated water; add nutrients and microorganisms (seed) • Measure dissolved oxygen (DO) levels over 5 days • Temperature 20° C • In dark (prevents algae from growing) • Final DO concentration must be > 2 mg/L • Need at least 2 mg/L change in DO over 5 days

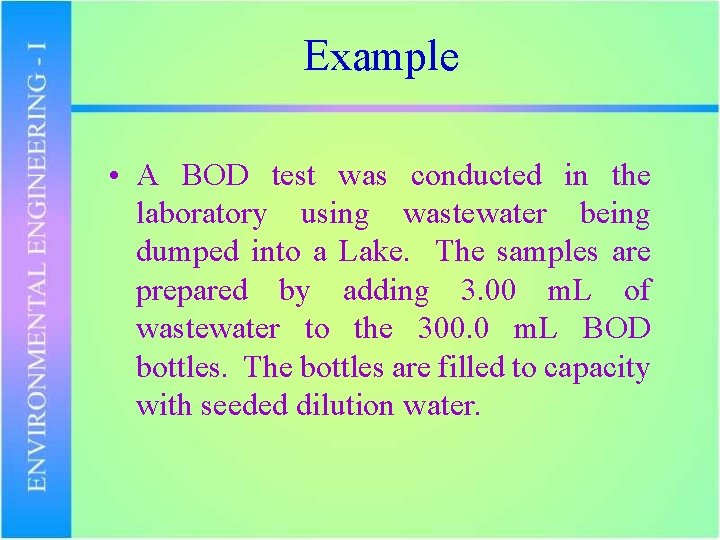
Example • A BOD test was conducted in the laboratory using wastewater being dumped into a Lake. The samples are prepared by adding 3. 00 m. L of wastewater to the 300. 0 m. L BOD bottles. The bottles are filled to capacity with seeded dilution water.

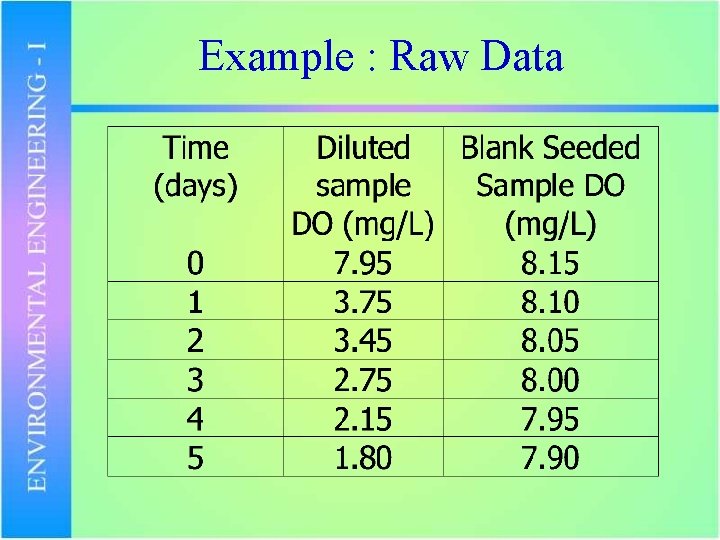
Example : Raw Data

Example : Calculations • What is the BOD 5 of the sample? • Plot the BOD with respect to time.

Example : Time – Concentration Plot

Modeling BOD as a First-order Reaction Organic matter oxidized Organic matter remaining

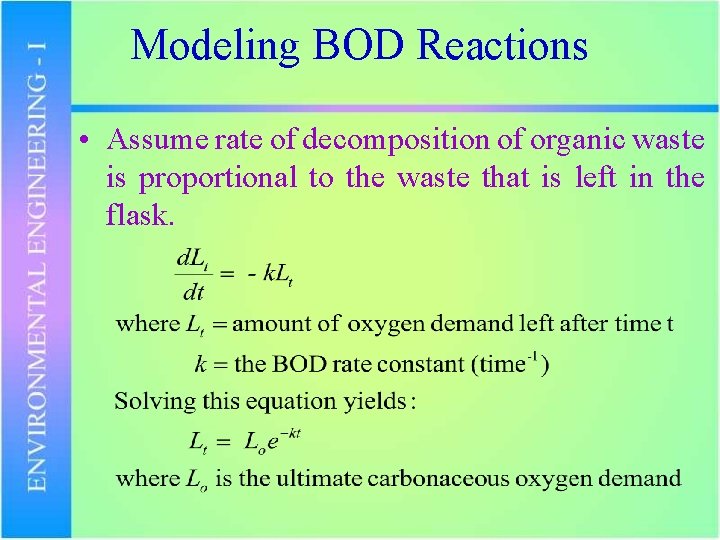
Modeling BOD Reactions • Assume rate of decomposition of organic waste is proportional to the waste that is left in the flask.

Lo Lo- Lt BOD exerted BODt Lt L remaining

Ultimate Biochemical Oxygen Demand Lt = amount of O 2 demand left in sample at time, t Lo = amount of O 2 demand left initially (at time 0, no DO demand has been exerted, so BOD = 0) At any time, Lo = BODt + Lt (that is the amount of DO demand used up and the amount of DO that could be used up eventually) Assuming that DO depletion is first order BODt = Lo(1 - e-kt)

Example • If the BOD 5 of a waste is 102 mg/L and the BOD 20 (corresponds to the ultimate BOD) is 158 mg/L, what is k?

Example (cont)

Biological Oxygen Demand: Temperature Dependence • Temperature dependence of biochemical oxygen demand !As temperature increases, metabolism increases, utilization of DO also increases kt = k 20 T-20 = 1. 135 if T is between 4 - 20 o. C = 1. 056 if T is between 20 - 30 o. C

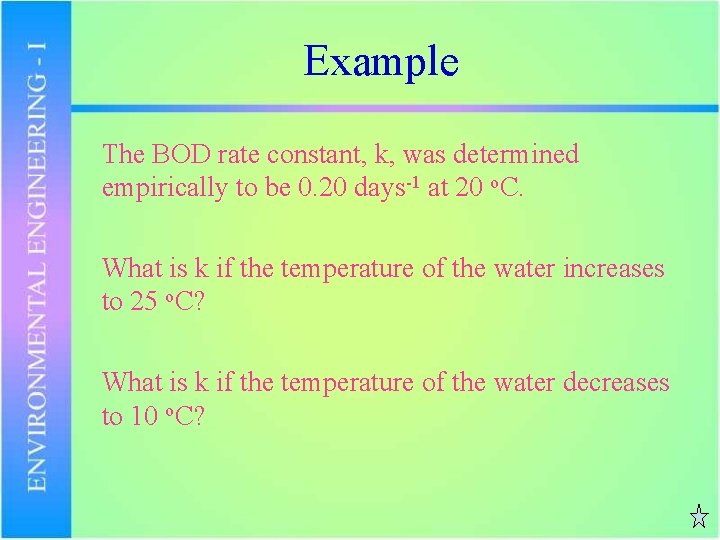
Example The BOD rate constant, k, was determined empirically to be 0. 20 days-1 at 20 o. C. What is k if the temperature of the water increases to 25 o. C? What is k if the temperature of the water decreases to 10 o. C?

Example

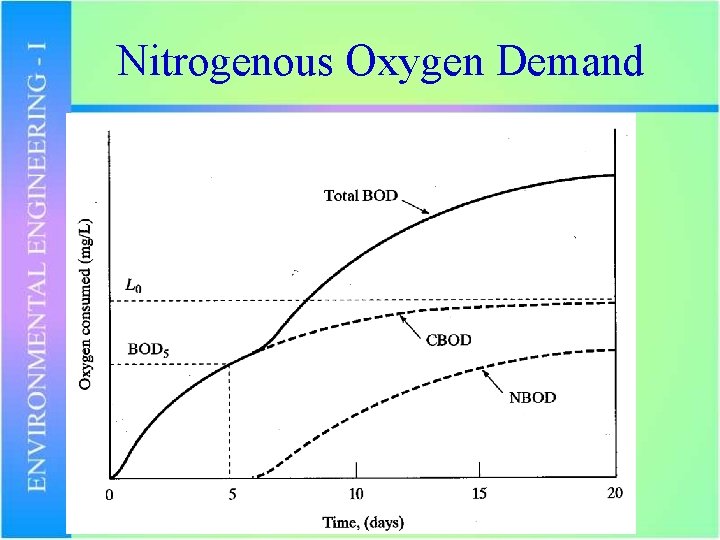
Nitrogenous Oxygen Demand • So far we have dealt only with carbonaceous demand (demand to oxidize carbon compounds) • Many other compounds, such as proteins, consume oxygen • Mechanism of reactions are different

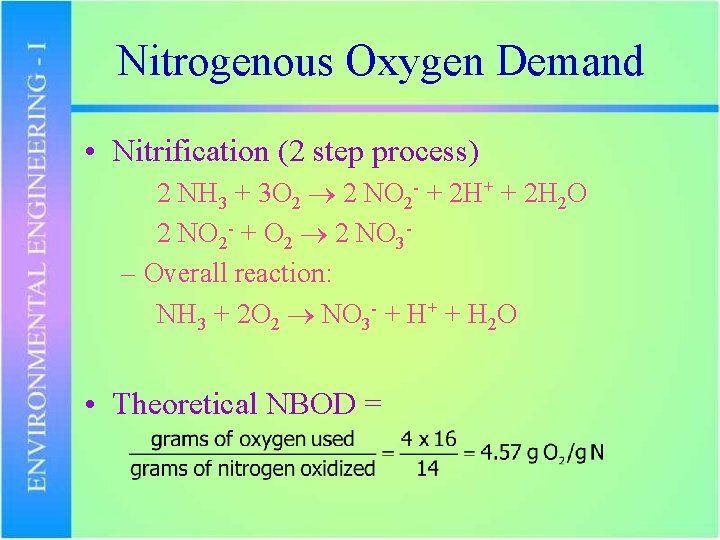
Nitrogenous Oxygen Demand • Nitrification (2 step process) 2 NH 3 + 3 O 2 2 NO 2 - + 2 H+ + 2 H 2 O 2 NO 2 - + O 2 2 NO 3– Overall reaction: NH 3 + 2 O 2 NO 3 - + H+ + H 2 O • Theoretical NBOD =

Nitrogenous Oxygen Demand

Nitrogenous oxygen demand • Untreated domestic wastewater ultimate-CBOD = 250 - 350 mg/L ultimate-NBOD = 70 - 230 mg/L Total Kjeldahl Nitrogen (TKN) = total concentration of organic and ammonia nitrogen in wastewater: 15 - 50 mg/L as N Ultimate NBOD 4. 57 x TKN

Other Measures of Oxygen Demand

Chemical Oxygen Demand • Chemical oxygen demand - similar to BOD but is determined by using a strong oxidizing agent to break down chemical (rather than bacteria) • Still determines the equivalent amount of oxygen that would be consumed • Value usually about 1. 25 times BOD

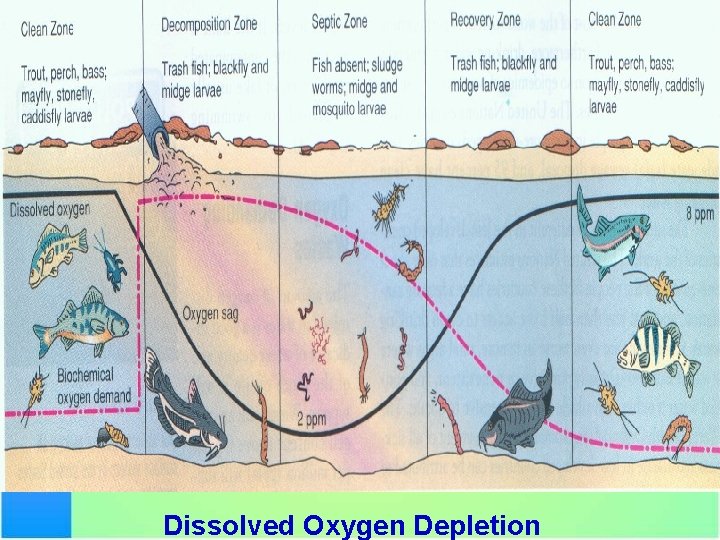
Water Quality Management in Rivers

Dissolved Oxygen Depletion

Dissolved Oxygen Sag Curve

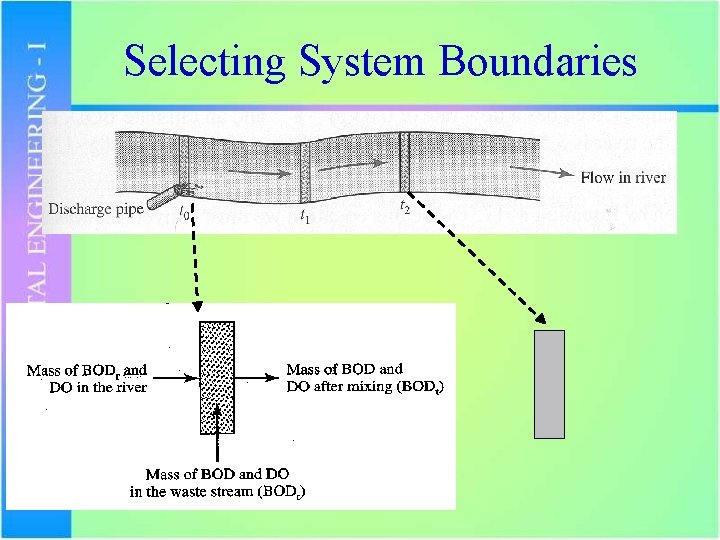
Mass Balance Approach • Originally developed by H. W. Streeter and E. B. Phelps in 1925 • River described as “plug-flow reactor” • Mass balance is simplified by selection of system boundaries • Oxygen is depleted by BOD exertion • Oxygen is gained through re-aeration

Steps in Developing the DO Sag Curve 1. Determine the initial conditions 2. Determine the re-aeration rate from stream geometry 3. Determine the de-oxygenation rate from BOD test and stream geometry 4. Calculate the DO deficit as a function of time 5. Calculate the time and deficit at the critical point

Selecting System Boundaries

Initial Mixing Qw = waste flow (m 3/s) DOw = DO in waste (mg/L) Lw = BOD in waste (mg/L) Qr = river flow (m 3/s) DOr = DO in river (mg/L) Lr = BOD in river (mg/L) Qmix = combined flow (m 3/s) DO = mixed DO (mg/L) La = mixed BOD (mg/L)

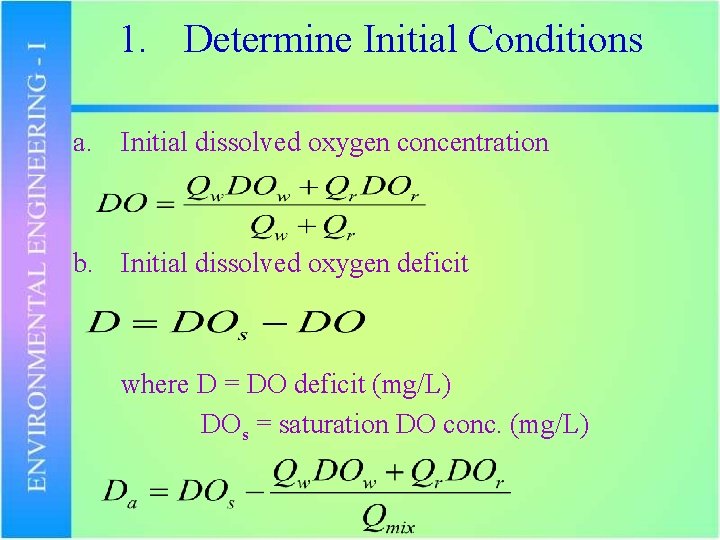
1. Determine Initial Conditions a. Initial dissolved oxygen concentration b. Initial dissolved oxygen deficit where D = DO deficit (mg/L) DOs = saturation DO conc. (mg/L)

1. Determine Initial Conditions c. DOsat is a function of temperature. Values can be found in Table. Initial ultimate BOD concentration

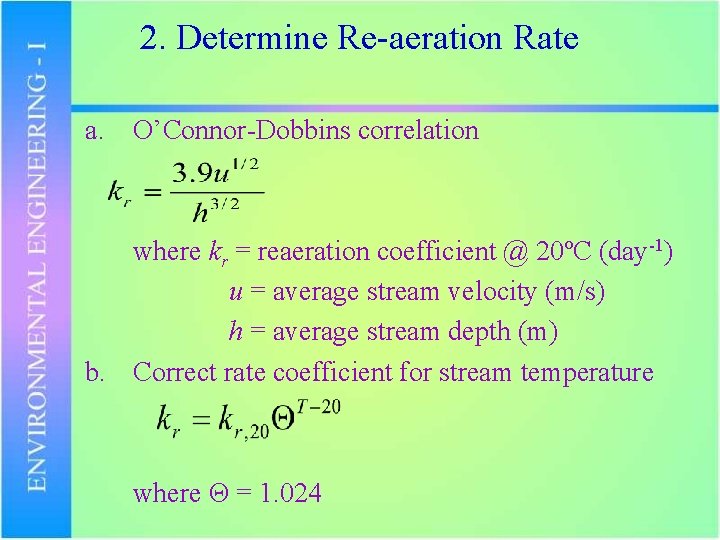
2. Determine Re-aeration Rate a. O’Connor-Dobbins correlation where kr = reaeration coefficient @ 20ºC (day-1) u = average stream velocity (m/s) h = average stream depth (m) b. Correct rate coefficient for stream temperature where Θ = 1. 024

Determine the De-oxygenation Rate a. rate of de-oxygenation = kd. Lt where kd = de-oxygenation rate coefficient (day-1) Lt = ultimate BOD remaining at time (of travel downstream) t b. If kd (stream) = k (BOD test) and

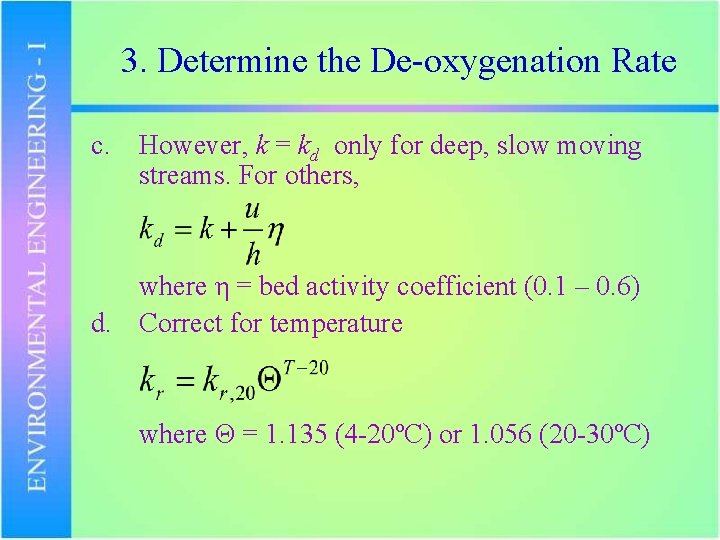
3. Determine the De-oxygenation Rate c. However, k = kd only for deep, slow moving streams. For others, where η = bed activity coefficient (0. 1 – 0. 6) d. Correct for temperature where Θ = 1. 135 (4 -20ºC) or 1. 056 (20 -30ºC)

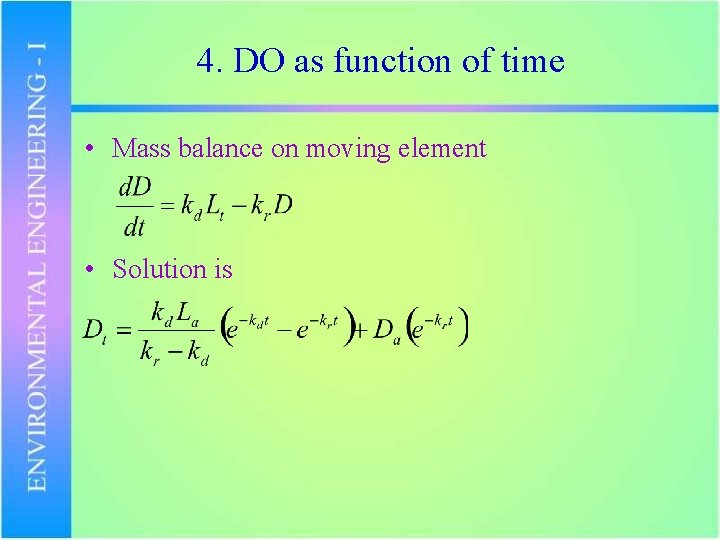
4. DO as function of time • Mass balance on moving element • Solution is

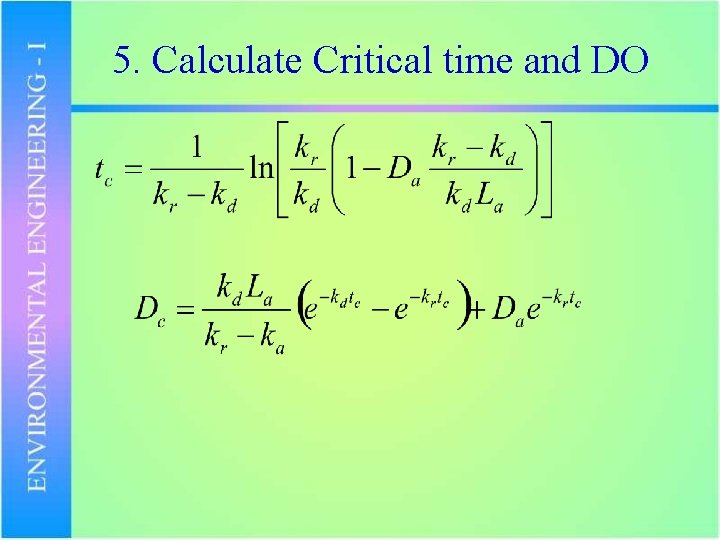
5. Calculate Critical time and DO
 Turbid definition
Turbid definition Why are some rivers in texas called “wrong way” rivers?
Why are some rivers in texas called “wrong way” rivers? Dissolved oxygen and chlorine molecules act as
Dissolved oxygen and chlorine molecules act as Principle of winkler's method
Principle of winkler's method Dissolved oxygen in air
Dissolved oxygen in air Winkler titration
Winkler titration What is dissolved oxygen
What is dissolved oxygen Water and water and water water
Water and water and water water Salt dissolved in water
Salt dissolved in water Chlorine and water equation
Chlorine and water equation Is sucrose soluble in water
Is sucrose soluble in water Sodium carbonate dissolved in water
Sodium carbonate dissolved in water Enthalpy specific heat equation
Enthalpy specific heat equation Water contracts (gets smaller) when it freezes.
Water contracts (gets smaller) when it freezes. Lettuce scheduling
Lettuce scheduling Objectives of irrigation
Objectives of irrigation Holy spirit rivers of living water
Holy spirit rivers of living water Lateral thinking brain teasers
Lateral thinking brain teasers Water quality management in india
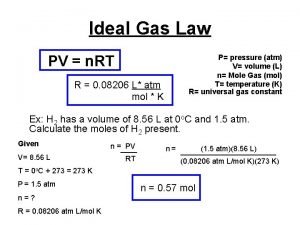
Water quality management in india Ideal gas law find n
Ideal gas law find n Mass of oxygen in water
Mass of oxygen in water Effect of ozone depletion on plants
Effect of ozone depletion on plants Baumeister self control
Baumeister self control Depletion formula
Depletion formula Ozone depletion effect on humans
Ozone depletion effect on humans Ece 424
Ece 424 Serhiy levkov
Serhiy levkov Depletion of animals
Depletion of animals Ch14 twitch
Ch14 twitch Journal entry for revaluation of assets
Journal entry for revaluation of assets Ozone layer
Ozone layer Ozone layer depletion introduction
Ozone layer depletion introduction Stratospheric ozone depletion
Stratospheric ozone depletion Chapter 11 depreciation
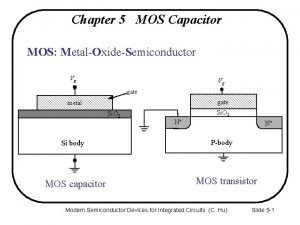
Chapter 11 depreciation Mosfet accumulation depletion inversion
Mosfet accumulation depletion inversion Depletion
Depletion Ozone depletion diagram
Ozone depletion diagram