Finding integrals is basically a reverse differentiation process. That is why integrals are also called anti-derivatives. Often the functions are straightforward and standard functions that can be integrated easily. It is easier to solve the combination of these functions using the properties of indefinite integrals. Sometimes functions are made up of compositions of two functions, these problems are harder to solve using the traditional way. In such cases, the U-substitution rule comes into play. Intuitively it is just the reverse of the chain rule. Let’s see this rule in detail.
Indefinite Integrals
Consider a derivative function f'(x) defined and differentiable in an interval. Indefinite Integrals or anti-derivatives allow us to reverse the process of differentiation and calculate the function F(x) whose derivative is given to us. There are infinitely many anti-derivatives for any given derivative. Mathematically integrals are denoted by the symbol ∫
F(x)= ∫ƒ(x) + C
Where C is an arbitrary constant. The table below shows some conventions and phrases used in the integration with their meaning.
| Symbol/Term/Meaning | Meaning |
| ∫f(x)dx | Integral of f with respect to x |
| f(x) in ∫f(x)dx | Integrand |
| x in ∫f(x)dx | Variable of integration |
| Integral of f(x) | A function such that F'(x) = f(x) |
It is essential to keep in mind the integrals of some standard functions so that the integration becomes simpler and less time-consuming. Integrals of some standard functions are shown below.
| Function | Integral |
| xn |  |
| sin(x) | -cos(x) |
| cos(x) | sin(x) |
| ex | ex |
| sec2(x) | tan(x) |
 | ln(x) |
Properties of Integrals
Property 1: ∫kf(x)dx= k∫f(x)dx
Property 2: ∫f(x)± g(x)dx= ∫f(x)dx± ∫g(x)dx
Property 3: ∫(f1(x)dx± f2(x)dx± f3(x)dx….)= ∫f1(x)dx± ∫f2(x)± ∫f3(x)dx…
U-Substitution Rule
Most often, integrals can be calculated using the properties and the formulas mentioned above. They allow us to calculate the simpler integrals in which integrands are usually a combination of some simple and standard functions. For example, consider the function, f(x) = cos(x) + 5, the integral of this function is easy and can be easily calculated using the properties mentioned above. But now consider another function, f(x) = sin(3x + 5). This function is a composition of two different functions, the integral for this function is not as easy as the previous one. Such integrals are solved using the U-substitution method.
∫f(g(x))g'(x)dx= ∫f(u)du
Here, u= g(x)
Consider an example to understand the rule.
f(x)= ∫2xcosx2dx
Notice that the part cos(x2) is a composite function. 2x is the derivative of the inner part x2. So, let’s say h(x) = x2 and w(x) = cos(x). So, we can assume that u(x) = x2 and w(x) = cos(x),

This is formulation of substitution. Consider u = x2

Thus, the function becomes,
f(x) = ∫cosu du
This can be solved using standard formulas,
f(x) = ∫cosu du = f(x) = sinu + C = f(x) = sinx2 + C
Let’s see some problems on this rule,
Related Articles:
Sample Problems
Question 1: Find the integral of the following function f(x),
f(x)= ∫10x(5x2)dx,
Solution:
Given f(x)= ∫10x(5x2)dx,
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx= F(x)= ∫10x(5x2)dx
Notice that the function is also a composite function,
Let u = 5x2. Differentiating the expression,

Using these results in the original integral equation,
F(x)= ∫f(x)dx= F(x)= ∫udu

Substituting the value of “u” in the above equation,
F(x) = 
Question 2: Find the integral of the following function f(x),
f(x) = 6x(3x2+ 5)
Solution:
Given f(x) = 6x(3x2+ 5),
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx= F(x)= ∫F(x)= ∫6x(3x2+ 5) dx
Notice that the function is also a composite function,
Let u = 3x2 + 5. Differentiating the expression,

Using these results in the original integral equation,
F(x)= ∫f(x)dx= F(x)= udu

Substituting the value of “u” in the above equation,
F(x) = 
Question 3: Find the integral of the following function f(x),
f(x)= ∫ (3x2+ 6x)(x3+ 3×2+ 5)
Solution:
Given f(x)= ∫ (3x2+ 6x)(x3+ 3×2+ 5),
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx= F(x)= ∫ (3x2+ 6x)(x3+ 3×2+ 5) dx
Notice that the function is also a composite function,
Let u = x3 + 3x2 + 5. Differentiating the expression,

Using these results in the original integral equation,
F(x)= ∫f(x)dx= F(x)= ∫udu

Substituting the value of “u” in the above equation,
F(x) = 
Question 4: Find the integral of the following function f(x),
f(x) = sin(x)cos(x)
Solution:
Given f(x) = sinxcosx,
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx= F(x)= ∫ sinxcosxdx
Notice that the function is also a composite function,
Let u = cos(x). Differentiating the expression,

Using these results in the original integral equation,
F(x)= ∫f(x)dx= F(x)= ∫ sin(x)cos(x)dx
F(x)= ∫-cos(x)(-sin(x))dx= F(x)= ∫-udu

Substituting the value of “u” in the above equation,
F(x) = 
Question 5: Find the integral of the following function f(x),
f(x)= ∫ 3x2cosx3
Solution:
Given f(x)= ∫ 3x2cosx3,
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx= F(x)= ∫ 3x2cosx3dx
Notice that the function is also a composite function,
Let u = x3. Differentiating the expression,
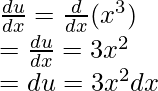
Using these results in the original integral equation,
F(x)= ∫f(x)dx
F(x)= ∫3x2cos(x)dx
F(x)= ∫cosu du
F(x) = sinu +C
Substituting the value of “u” in the above equation,
F(x) = sin(u) + C
⇒F(x) = sin(x3) + C
Question 6: Find the integral of the following function f(x),
f(x) = ∫3x2cosx3
Solution:
Given f(x)= ∫3x2cosx3,
Let F(x) be the integral of the function f(x),
F(x)= ∫f(x)dx
F(x)= ∫3x2cosx3dx
Notice that the function is also a composite function,
Let u = x3. Differentiating the expression,
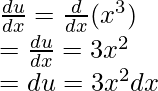
Using these results in the original integral equation,
F(x)= ∫f(x)dx
F(x)= ∫3x2cos(x)dx
F(x)= ∫cosu du
F(x)= sin(u)+ C
Substituting the value of “u” in the above equation,
F(x) = sin(u) + C
⇒F(x) = sin(x3) + C
Integration by U-substitution Worksheet
1: Evaluate the integral:
2: Evaluate the integral: 
3: Evaluate the integral: 
4: Evaluate the integral: 
5: Evaluate the integral: 
6: Find the integral of the following function f(x),
f(x) = sin(x)cos(x)
7: Find the integral of the following function f(x),
f(x) =cos(x)
8: Find the integral of the following function f(x),
f(x) = sin(x)
9: Find the integral of the following function f(x),
f(x) = sin2(x)cos(x)
10: Find the integral of the following function f(x),
f(x) = sin3(x)cos2(x)
Summary
Integration by U-Substitution is a technique used to simplify integrals by substituting a part of the integrand with a new variable, uuu, to make the integral easier to solve. This method is particularly useful when dealing with composite functions or when the integrand is a product of functions.
FAQs on Integration by U-substitution
What is the purpose of U-Substitution?
U-Substitution simplifies integrals by transforming them into a form that is easier to integrate. It is particularly useful for integrals involving composite functions or when the integrand has a specific structure that suggests a substitution.
How do you choose the substitution?
Choose uuu to be a function within the integrand that, when substituted, simplifies the integral. Common choices are functions inside parentheses, exponents, or arguments of trigonometric functions.
Can U-Substitution be used for definite integrals?
Yes, U-Substitution can be used for definite integrals. When using U-Substitution for definite integrals, also change the limits of integration to reflect the new variable uuu. After integrating, substitute back to the original variable to find the final result.
What should you do if the substitution does not simplify the integral?
If the substitution does not simplify the integral, you might need to try a different substitution or use another method of integration, such as integration by parts or partial fractions.