SlideShare a Scribd company logo

11X1 t01 08 completing the square (2012)

•
•
N
Nigel Simmons

The document discusses the process of completing the square to solve quadratic equations. It shows how to complete the square for a general quadratic equation of the form ax2 + bx + c = 0 by grouping like terms and factorizing into a perfect square form. Examples are worked through, including solving the specific equations x2 + 6x - 7 = 0, x2 - 6x + 6 = 0.

1 of 20
Downloaded 33 times
Completing the Square
Completing the Square e.g. (i ) x 2  6 x  7  0
Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant
Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared
Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square
Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square x  3  4
Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square x  3  4 x  3  4 x  7 or x  1
(ii ) ax 2  bx  c  0
(ii ) ax 2  bx  c  0 b c x2  x   0 a a
(ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a
(ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a 
(ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2
(ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2 b b 2  4ac x  2a 2a
(ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2 b b 2  4ac x  2a 2a b  b 2  4ac x 2a
(iii ) x 2  6 x  6  0
(iii ) x 2  6 x  6  0  x  3 0 2
(iii ) x 2  6 x  6  0  x  3 3  0 2
(iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0
(iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0 x  3  3 or x  3  3
(iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0 x  3  3 or x  3  3 Exercise 1I; 1adh, 2ch, 3adg, 4bdfh, 5bdf, 6adg, 7bc, 8*

More Related Content

11X1 t01 08 completing the square (2012)

  • 2. Completing the Square e.g. (i ) x 2  6 x  7  0
  • 3. Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant
  • 4. Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared
  • 5. Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square
  • 6. Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square x  3  4
  • 7. Completing the Square e.g. (i ) x 2  6 x  7  0 x2  6x  7 move the constant x 2  6 x  32  7  32 add half the coefficient of ‘x’ squared x 2  6 x  9  16  x  3  16 2 factorise to a perfect square x  3  4 x  3  4 x  7 or x  1
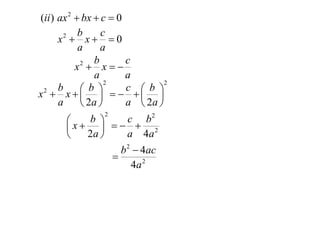
  • 8. (ii ) ax 2  bx  c  0
  • 9. (ii ) ax 2  bx  c  0 b c x2  x   0 a a
  • 10. (ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a
  • 11. (ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a 
  • 12. (ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2
  • 13. (ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2 b b 2  4ac x  2a 2a
  • 14. (ii ) ax 2  bx  c  0 b c x2  x   0 a a b c x  x 2 a a 2 2 x2  x         b b c b     a  2a  a  2a  2 x b  c  b 2    2a  a 4a 2 b 2  4ac  4a 2 b b 2  4ac x  2a 2a b  b 2  4ac x 2a
  • 15. (iii ) x 2  6 x  6  0
  • 16. (iii ) x 2  6 x  6  0  x  3 0 2
  • 17. (iii ) x 2  6 x  6  0  x  3 3  0 2
  • 18. (iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0
  • 19. (iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0 x  3  3 or x  3  3
  • 20. (iii ) x 2  6 x  6  0  x  3 3  0 2  x  3  3  x  3  3   0 x  3  3 or x  3  3 Exercise 1I; 1adh, 2ch, 3adg, 4bdfh, 5bdf, 6adg, 7bc, 8*