
Answer
475.8k+ views
Hint: First we write the general equation of a line which is $ y=mx+c $ , where $ m= $ slope of a line and $ c= $ constant. Then, we find the value of slope and value of constant to write the equation of $ x $ axis.
Complete step-by-step answer:
We know that the general equation of a line is given by $ y=mx+c $ , where $ m= $ slope of a line and $ c= $ constant. This form is also called the slope-intercept form of a line.
Also, we know that the slope is defined as $ m=\tan \theta $ .
Where, $ \theta $ is the angle made by line with the positive direction of the $ x $ axis.
We know that $ x $ axis is actually a straight line. So, we get that the value of $ \theta $ will be $ 0 $ . Also, we know that $ \tan 0{}^\circ =0 $ .
So, we can substitute this and get the value of slope as $ m=0 $
Now, the value of $ c $ will be zero, because we know that the $ x $ axis is passes through origin and is parallel to itself at a distance $ 0 $ from it. So, the points will be $ \left( 0,0 \right) $ .
Now, when we put the value of $ m $ and $ c $ in the general equation of line, we get
$ \begin{align}
& y=mx+c \\
& \Rightarrow y=0\times x+0 \\
& \Rightarrow y=0 \\
\end{align} $
So, the equation of $ x $ axis is $ y=0 $
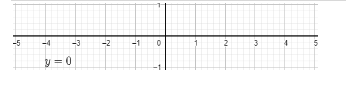
We can also get the plot the equation and get the x-axis as shown below,
Note: The general equation of a line in three dimensional is given by the equation \[\dfrac{x-{{x}_{1}}}{a}=\dfrac{y-{{y}_{1}}}{b}=\dfrac{z-{{z}_{1}}}{c}=r\]
When we have to write the equation of $ x $ axis, the $ y $ and $ z $ coordinates would be zero, so the equation of $ x $ axis will be $ \dfrac{X}{1}=\dfrac{Y}{0}=\dfrac{Z}{0} $ .
Complete step-by-step answer:
We know that the general equation of a line is given by $ y=mx+c $ , where $ m= $ slope of a line and $ c= $ constant. This form is also called the slope-intercept form of a line.
Also, we know that the slope is defined as $ m=\tan \theta $ .
Where, $ \theta $ is the angle made by line with the positive direction of the $ x $ axis.
We know that $ x $ axis is actually a straight line. So, we get that the value of $ \theta $ will be $ 0 $ . Also, we know that $ \tan 0{}^\circ =0 $ .
So, we can substitute this and get the value of slope as $ m=0 $
Now, the value of $ c $ will be zero, because we know that the $ x $ axis is passes through origin and is parallel to itself at a distance $ 0 $ from it. So, the points will be $ \left( 0,0 \right) $ .
Now, when we put the value of $ m $ and $ c $ in the general equation of line, we get
$ \begin{align}
& y=mx+c \\
& \Rightarrow y=0\times x+0 \\
& \Rightarrow y=0 \\
\end{align} $
So, the equation of $ x $ axis is $ y=0 $
We can also get the plot the equation and get the x-axis as shown below,

Note: The general equation of a line in three dimensional is given by the equation \[\dfrac{x-{{x}_{1}}}{a}=\dfrac{y-{{y}_{1}}}{b}=\dfrac{z-{{z}_{1}}}{c}=r\]
When we have to write the equation of $ x $ axis, the $ y $ and $ z $ coordinates would be zero, so the equation of $ x $ axis will be $ \dfrac{X}{1}=\dfrac{Y}{0}=\dfrac{Z}{0} $ .



