Tutors Answer Your Questions about Trigonometry-basics (FREE)
Question 1198808: The bearing from A to B is S 27⁰ E, and the bearing from B to C is N 63⁰ E. A ship traveling at 12 nautical miles per hour needs 1.25 hours to go from A to B and 1.75 hours to go from B to C. Find the bearing, to the nearest tenth, from B to A.
Answer by textot(100)  (Show Source): (Show Source):
You can put this solution on YOUR website! **1. Understand Bearings**
* Bearings are measured clockwise from North.
* S 27° E means 27° East of South.
* N 63° E means 63° East of North.
**2. Find the Bearing from B to A**
* **Bearing from A to B:** S 27° E
* This is 27° clockwise from South.
* To find the bearing from B to A, we need to find the opposite direction.
* **Bearing from B to A:**
* 180° (South to North) + 27° = 207°
* This is 207° clockwise from North, which can be written as **S 73° W**
**Therefore, the bearing from B to A is S 73° W.**
**Note:**
* The given information about the ship's speed and travel times is not necessary to solve this problem.
* The bearing from B to A depends solely on the directions of travel between the points.
Question 1204330: Find the sum
𝑆 = cos(𝑥 + 𝜃) + cos(2𝑥 + 3𝜃) + cos(3𝑥 + 5𝜃) + cos(4𝑥 + 7𝜃) + ⋯ + cos(40𝑥 + 79𝜃),
and express your answer as a product and quotient of trigonometric functions.
Answer by ElectricPavlov(30)  (Show Source): (Show Source):
You can put this solution on YOUR website! Certainly, let's find the sum of the given series.
**1. Observation**
The given series exhibits a pattern in the arguments of the cosine function:
* The coefficient of 'x' increases by 1 in each term.
* The coefficient of 'θ' increases by 2 in each term.
**2. Utilize the Product-to-Sum Formula**
We can use the product-to-sum trigonometric identity to express each term of the series as a product of sines and cosines:
cos(A) + cos(B) = 2 * cos[(A + B)/2] * cos[(A - B)/2]
**3. Apply the Product-to-Sum Formula to the Series**
* Let's apply the product-to-sum formula to the first two terms:
cos(x + θ) + cos(2x + 3θ)
= 2 * cos[(x + θ + 2x + 3θ)/2] * cos[(x + θ - 2x - 3θ)/2]
= 2 * cos[(3x + 4θ)/2] * cos[(-x - 2θ)/2]
= 2 * cos[(3x + 4θ)/2] * cos[(x + 2θ)/2]
* Continue applying the product-to-sum formula to the subsequent pairs of terms. You'll notice a pattern emerging in the arguments of the cosine functions.
**4. Generalize the Pattern**
* By carefully observing the pattern after applying the product-to-sum formula repeatedly, you'll find that the sum 'S' can be expressed as a product of a series of cosine terms.
**5. Simplify and Express as Product and Quotient**
* After obtaining the product of cosine terms, you might be able to further simplify the expression by using other trigonometric identities or by grouping terms.
* The final expression for 'S' will be in the form of a product of trigonometric functions divided by another trigonometric function.
**Important Notes:**
* The exact form of the final expression will depend on the specific pattern observed after applying the product-to-sum formula repeatedly.
* This process can be quite intricate.
**To get the precise answer, I recommend:**
1. **Apply the product-to-sum formula** to a few more terms of the series to identify the pattern clearly.
2. **Use a computer algebra system (like Mathematica or Wolfram Alpha)** to perform the symbolic calculations and simplify the resulting expression.
I hope this approach helps! Let me know if you have any further questions or if you'd like me to assist with the calculations for a specific number of terms.
Question 1209251: A Ferris wheel with a radius of 45 meters is elevated on a platform 5 meters above the ground. One full rotation takes 40 minutes. The height h(t) of a passenger is given by h(t) = 45sin(pi/20t) + 50. What is the first time t (in minutes) when the height is 72.5 meters?
Answer by math_tutor2020(3700)  (Show Source): (Show Source):
You can put this solution on YOUR website!
Let w = sin(pi*t/20)
45sin(pi*t/20) + 50 becomes 45w + 50
Solving 45w + 50 = 72.5 leads to w = 0.5 and it leads back to sin(pi*t/20) = 0.5
If sin(x) = 0.5 in radian mode, then x = pi/6 and x = 5pi/6 are two possibilities when considering the interval 
This is something to memorize since it comes up a lot in trig. Alternatives are to use a reference sheet, unit circle, or a calculator.
We use the smaller of those x values since we're interested when the passenger reaches 72.5 meters the first time. If we changed "first time" to "second time", then we'd use 5pi/6 instead.
So,
sin(pi*t/20) = 0.5
sin(pi*t/20) = sin(pi/6)
pi*t/20 = pi/6
t/20 = 1/6
t = (1/6)*20
t = 20/6
t = 10/3
t = 3.3333... where the '3's go on forever
This is the number of minutes it takes to arrive at the first instance of being 72.5 meters above the ground.
Round that decimal value however your teacher instructs.
Or you can stick with the fraction form.
Extra info:
(10/3) minutes = (10/3)*60 = 200 seconds
200 seconds = 180 seconds + 20 seconds = 3 minutes + 20 seconds
Question 1209173: sandy is trying to measure the height of a nearby flagpole using a mirror. The mirror is 6m away from the flagpole and 2 m away from sandy. If sandy is 1.5m tall how tall is the flagpole?
Found 2 solutions by ikleyn, timofer:
Answer by ikleyn(51772)   (Show Source): (Show Source):
Answer by timofer(70)  (Show Source): (Show Source):
Question 1208979: A boy flying a kite lets out 300 feet of string which makes an angle of 38° with the ground. Assuming that the string is straight, how high above the ground is the kite? Round to the nearest foot
Answer by ikleyn(51772)   (Show Source): (Show Source):
Question 1208915: Solve for x.
3sec x + 5tan x = sin x
Answer by mccravyedwin(315)   (Show Source): (Show Source):
Question 1208913: Solve for x.
3csc x - [1/cos x] = 0
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Solve for x.
3csc x - [1/cos x] = 0
~~~~~~~~~~~~~~~~~~~~~~~
You want to solve
 - -  = 0.
The domain of this equation are all real numbers except of x = = 0.
The domain of this equation are all real numbers except of x =  , where sin(x) = 0 or cos(x) = 0.
In the domain, multiply both sides by sin(x).
Since sin(x) =/= 0, you get an equivalent equation
3 - , where sin(x) = 0 or cos(x) = 0.
In the domain, multiply both sides by sin(x).
Since sin(x) =/= 0, you get an equivalent equation
3 -  = 0,
which is the same as
3 = = 0,
which is the same as
3 =  ,
or
tan(x) = 3.
Hence, the general solution to the given equation is the set x = arctan(3) + ,
or
tan(x) = 3.
Hence, the general solution to the given equation is the set x = arctan(3) +  = 1.24904577 + = 1.24904577 +  radians, k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x = arctan(3) + radians, k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x = arctan(3) +  = 1.24904577 + = 1.24904577 +  radians, k = 0, +/-1, +/-2, . . . radians, k = 0, +/-1, +/-2, . . .
Solved.
Question 1208914: Solve for x.
5sec x + [tan x/cos x] = 0
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Solve for x.
5sec x + [tan x/cos x] = 0
~~~~~~~~~~~~~~~~~~~~~~~
You want to solve
 + +  = 0.
The domain of this equation are all real numbers except of x = = 0.
The domain of this equation are all real numbers except of x =  ,
where cos(x) = 0.
In the domain, multiply both sides by cos(x).
Since cos(x) =/= 0, you get an equivalent equation
5 + tan(x) = 0.
which is the same as
tan(x) = -5.
Hence, x = arctan(-5) + ,
where cos(x) = 0.
In the domain, multiply both sides by cos(x).
Since cos(x) =/= 0, you get an equivalent equation
5 + tan(x) = 0.
which is the same as
tan(x) = -5.
Hence, x = arctan(-5) +  = -arctan(5) + = -arctan(5) +  = -1.37340077 + = -1.37340077 +  radians, k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x = -arctan(5) + radians, k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x = -arctan(5) +  = -1.37340077 + = -1.37340077 +  radians, k = 0, +/-1, +/-2, . . . radians, k = 0, +/-1, +/-2, . . .
Solved.
Question 1208912: Solve for x.
2sinx + 2tanx = 0
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Solve for x.
2sinx + 2tanx = 0
~~~~~~~~~~~~~~~~~~~~~~~
You want to solve
2sin(x) + 2tan(x) = 0.
The domain of this equation are all real numbers except of x =  , where cos(x) = 0.
Rewrite in the form
2sin(x) + , where cos(x) = 0.
Rewrite in the form
2sin(x) +  = 0.
In the domain, multiply both sides by = 0.
In the domain, multiply both sides by  .
Since cos(x) =/= 0, you will get an equivalent equation
sin(x)*cos(x) + sin(x) = 0.
Factor
sin(x)*(cos(x) + 1) = 0.
So, either sin(x) = 0, giving the solutions x = .
Since cos(x) =/= 0, you will get an equivalent equation
sin(x)*cos(x) + sin(x) = 0.
Factor
sin(x)*(cos(x) + 1) = 0.
So, either sin(x) = 0, giving the solutions x =  , k = 0, +/-1, +/-2, . . . (1)
or cos(x) + 1 = 0, cos(x) = -1, giving x = , k = 0, +/-1, +/-2, . . . (1)
or cos(x) + 1 = 0, cos(x) = -1, giving x =  , k = 0, +/-1, +/-2, . . . (2)
The set (2) is part of set (1) - so, the general solution to the given equation is the set
x = , k = 0, +/-1, +/-2, . . . (2)
The set (2) is part of set (1) - so, the general solution to the given equation is the set
x =  , k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x = , k = 0, +/-1, +/-2, . . .
ANSWER. The general solution to the given equation is the set x =  , k = 0, +/-1, +/-2, . . . , k = 0, +/-1, +/-2, . . .
Solved.
Question 1208905: Solve for x over the domain of -2pi <= x <= 2pi and find all 5 solutions.
2tanx + secx = 1
I have gotten to the answer of (sinx)(5sinx +4)=0 but this yields 7 solutions.
Thank you.
Answer by greenestamps(13008)   (Show Source): (Show Source):
You can put this solution on YOUR website!
In transforming the given equation into an equation in sin(x), you had to square both sides of the equation at some point. That can always introduce extraneous roots.
The given equation is true at both endpoints of the prescribed interval, so your equation in sin(x) yields 9 roots, not 7.
At 4 of those 9 roots, the value of the given expression 2tan(x)+sec(x) is equal to -1 instead of +1. (-1)^2 = 1, so those extraneous roots were introduced when you squared both sides of the equation.
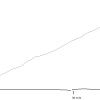
Here is a graph showing the original equation (red) and your equation in sin(x) (green). Also shown are graphs of the constants 1 and -1.
You can see that, at 5 of the 9 points where the equation in sin(x) is satisfied (value of green graph is 0), the value of the given equation is equal to 1 (red graph intersects blue graph). Those points represent the solutions to the original equation.
But you can also see that, at the other 4 of the 9 points where the equation in sin(x) is satisfied (value of green graph is 0), the value of the given equation is -1 instead of 1 (red graph intersects purple graph).

Question 1208900: 1. A flagpole 4m high stands at the top of a pedestal 2.5m high located at one side of a pathway. At the opposite side of the pathway directly facing the flagpole, the flagpole subtends the same angle as the pedestal. What is the width of the pathway?
2. From a window
3. An Air Force
a. What is
b. How high
c. How high
4. The angle
a. The distance
b. The distance
c. The height
5. The angle
Found 2 solutions by ikleyn, KMST:
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
One and ONLY ONE problem/question per post.
It is the RULE, the POLICY and the REQUIREMENT of this forum.
It is written in this page
https://www.algebra.com/tutors/students/ask.mpl?action=ask_question&topic=Equations&return_url=http://www.algebra.com/algebra/homework/equations/
from which you post your problems.
It is assumed that you read these rules before posting.
It is also assumed that you do understand what is written in that page and follow the rules.
Those who violate them, work against their own interests.
Answer by KMST(5326)   (Show Source): (Show Source):
Question 1208881: If sin 40° + sin 20° = sin 𝜃, find the value of 𝜃.
Found 2 solutions by mccravyedwin, ikleyn:
Answer by mccravyedwin(315)   (Show Source): (Show Source):
Answer by ikleyn(51772)   (Show Source): (Show Source):
Question 1208813: Solve for x.
(sin x + cos x)/(1 - tan x) = 0
Found 2 solutions by ikleyn, AnlytcPhil:
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Solve for x: (sin x + cos x)/(1 - tan x) = 0
~~~~~~~~~~~~~~~~~~~~
The solution in the post by Edwin is incomplete.
I came to bring a complete solution.
The domain (the set of real numbers, where left side of the equation
is defined) is tan(x) =/= 1.
So, the prohibited values of x are  , k = 0, _/-1, +/-2, . . .
We are looking for solutions of the given equation that are in its domain.
In the domain, the given equation is equivalent to
sin(x) + cos(x) = 0, (1)
or
sin(x) = -cos(x). (2)
The solutions to this equation can not be with cos(x) = 0 (since then sin(x) = 1,
and this equation is not held) .
Therefore, we can divide both sides of equation (2) by cos(x). We get then
tan(x) = -1.
The solutions to this equation are
x = , k = 0, _/-1, +/-2, . . .
We are looking for solutions of the given equation that are in its domain.
In the domain, the given equation is equivalent to
sin(x) + cos(x) = 0, (1)
or
sin(x) = -cos(x). (2)
The solutions to this equation can not be with cos(x) = 0 (since then sin(x) = 1,
and this equation is not held) .
Therefore, we can divide both sides of equation (2) by cos(x). We get then
tan(x) = -1.
The solutions to this equation are
x =  , or 135° + 180°*k, k = 0, +/-1, +/-2, . . .
ANSWER. The solutions to the given equation are x = , or 135° + 180°*k, k = 0, +/-1, +/-2, . . .
ANSWER. The solutions to the given equation are x =  , or 135° + 180°*k, k = 0, +/-1, +/-2, . . . , or 135° + 180°*k, k = 0, +/-1, +/-2, . . .
Solved.
Answer by AnlytcPhil(1791)   (Show Source): (Show Source):
Question 1208769: Prove that: 1/(cosec A - cot A) - 1/sin A = 1/sin A - 1/(cosec A + cot A)
Answer by Edwin McCravy(19937)   (Show Source): (Show Source):
Question 1208766: Solve sin^2 x + sin x + 4 = 0 for x.
Found 2 solutions by math_tutor2020, Shin123:
Answer by math_tutor2020(3700)  (Show Source): (Show Source):
You can put this solution on YOUR website!
Let w = sin(x)
The original equation given to you becomes w^2 + w + 4 = 0
You should find the discriminant is d = b^2-4ac = 1^2 - 4*1*4 = -15
Because d < 0, it means w^2 + w + 4 = 0 has two complex number solutions of the form a+bi, where i = sqrt(-1)
This must mean that the original equation also has complex number solutions of the form a+bi.
Therefore, sin^2(x)+sin(x)+4 = 0 does not have any real number solutions.
Here's a graph of y = ( sin(x) )^2 + sin(x) + 4.

The curve never touches the x axis.
--------------------------------------------------------------------------
Another way to look at it.
Find the vertex of w^2 + w + 4. I'll let the student handle the scratch work, but you should find the lowest point for w^2 + w + 4 is when w = -1/2
Plug w = -1/2 into w^2 + w + 4 and you'll get 15/4 = 3.75
This shows that the smallest w^2 + w + 4 can get is 3.75, which unfortunately is above 0.
Meaning that w^2 + w + 4 will never be 0 no matter what you pick for w.
Consequently, no matter what real number you pick for x, sin^2(x)+sin(x)+4 will never be 0 either.
Answer by Shin123(626)   (Show Source): (Show Source):
Question 1208768: Prove (1 - sin A)/(1 + sin A) = (sec A - tan A)^2
Answer by math_tutor2020(3700)  (Show Source): (Show Source):
Question 1208767: Simplify sec x/tan x.
Use trigonometric identities.
Answer by ikleyn(51772)   (Show Source): (Show Source):
Question 1208765: Solve sin x + cos x = 1 for x.
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Solve sin x + cos x = 1 for x.
~~~~~~~~~~~~~~~~~~~~~~~
We start from
sin x + cos x = 1 for x. (1)
Square both sides
sin^2(x) + cos^2(x) + 2*sin(x)*cos(x) = 1.
Replace sin^2(x) + cos^2(x) by 1 (according the Pythagorean theorem)
1 + 2*sin(x)*cos(x) = 1.
Cancel "1" in both sides and write in the form
sin(2x) = 0.
Hence, 2x =  .
It implies x = .
It implies x =  , n = 0, +/-1, +/-2, . . . (2)
We squared the original equation - so, erroneous and excessive roots could arise.
Therefore, we should check the roots (2).
Checking shows that only x with n = 4k and n = 4k+1 satisfy the original equation.
It gives the ANSWER : the solutions are x = , n = 0, +/-1, +/-2, . . . (2)
We squared the original equation - so, erroneous and excessive roots could arise.
Therefore, we should check the roots (2).
Checking shows that only x with n = 4k and n = 4k+1 satisfy the original equation.
It gives the ANSWER : the solutions are x =  and x = and x =  , k = 0, +/-1, +/-2, . . . , k = 0, +/-1, +/-2, . . .
Solved.
Question 1208644: Royal Cruise and Carnival Cruise start at island point A. Royal sails 35 km on a bearing N 40 E to island B. Carnival sails in the direction S 30 W to island C. The distance from A to C is 70 km. How far is it from B to C?
Found 2 solutions by ikleyn, math_tutor2020:
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Consider triangle ABC.
Its side AB is 35 kilometers long..
Its side AC is 70 kilometers long.
The angle A is 50 degrees + 90 degrees + 30 degrees = 170 degrees.
To find the length of the side BC, use the cosine law
BC =  = =  = 104.645 km (rounded).
ANSWER. The distance from A to C is about 104.6 kilometers. = 104.645 km (rounded).
ANSWER. The distance from A to C is about 104.6 kilometers.
Solved.
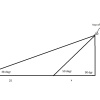
Answer by math_tutor2020(3700)  (Show Source): (Show Source):
You can put this solution on YOUR website!
Answer: 104.644914 km (approximate)
Explanation
Draw an xy axis.
Let's place point A at the origin (0,0).
Island B is somewhere in the northeast quadrant (aka upper right corner).
This is because notation like "N 40 E" means we face directly north and then turn 40 degrees toward the east.
Draw a segment to connect points A and B.
This segment will be the hypotenuse of a right triangle such that its vertical leg is on top of the positive y axis.
The horizontal leg is not on the x axis. Refer to the diagram shown below.
The vertical leg is labeled as dy.
The horizontal leg is labeled as dx.
The angle theta (symbol  ) is the acute angle between the hypotenuse and vertical leg. ) is the acute angle between the hypotenuse and vertical leg.
r = hypotenuse = distance the boat travels from A to B

If you know your trig ratios, then you should realize that:
dx = r*sin(theta)
dy = r*cos(theta)
Those formulas are very useful to determine the location of island B when A = (0,0).
The dx and dy are the components of the translation vector to get from A to B.
You should find that:dx = r*sin(theta) = 35*sin(40) = 22.497566
dy = r*cos(theta) = 35*cos(40) = 26.811556 Each result is approximate. Your calculator needs to be in degree mode.
Point B is at the approximate location B = (22.497566, 26.811556) when A = (0,0).
-----------
We'll use the same idea, more or less, to figure out where point C is located on the xy grid.
Let's calculate the dx and dy values when the Carnival boat sails r = 70 kilometers and the angle mentioned is theta = 30.
We'll worry about the southwest direction later.dx = r*sin(theta) = 70*sin(30) = 35
dy = r*cos(theta) = 70*cos(30) = 60.621778 approximately Now if Carnival was sailing northeast, then we wouldn't make any adjustments to the signs of dx and dy.
However, it's instead going southwest, so we need to flip the sign of each.
The location of island C is roughly (-35, -60.621778) when A = (0,0).
I suppose you could have this rough sketch on a notecard (or have it memorized)

Or just remember to use common sense intuition when figuring out the positive or negative signs.
----------
Summary so far:
Islands B and C are at these approximate locations when A = (0,0)- B = (22.497566, 26.811556)
- C = (-35, -60.621778)
The next step from here is to use the distance formula to figure out how far it is from B to C.
Distance formula: 
I'll let the student do the scratch work.
You should get the approximate answer of 104.644914 km.
The answer will slightly vary depending how your teacher wants you to round.
I used GeoGebra to verify the answer.
Here is the specific worksheet I made for this problem. If the page is blank, then hover your mouse over any part of the box. A spiral arrow should show up. Click on it to have it refresh the page.
Question 1208456: 7. P is the centre of the upper face of the rectangular block shown in the figure. Calculate
(a) PẬC,
(b) PÂB.
A 3D cuboid figure is given in which its given that ABCD is the base of the figure its length AD=BC= 16cm, its breadth AB=DC=12cm, and given its height from every corner of base to the upper portion of the 3D figure is 5cm. Find the angles in question above.
Answer by AnlytcPhil(1791)   (Show Source): (Show Source):
Question 1208450: If cot(theta) is undefined, and cos(theta) < 0, what are the values of the six trigonometric functions?
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
If cot(theta) is undefined, and cos(theta) < 0, what are the values
of the six trigonometric functions?
~~~~~~~~~~~~~~~~~~
Then theta =  , or 180 degrees.
sin(theta) = 0
cos(theta) = -1
tan(theta) = 0
cot(theta) is undefined
sec(theta) is -1
csc(theta) is undefined. , or 180 degrees.
sin(theta) = 0
cos(theta) = -1
tan(theta) = 0
cot(theta) is undefined
sec(theta) is -1
csc(theta) is undefined.
-------------------
It's like asking about the multiplication table.
A good student's answers should bounce off like ping-pong balls off a wall.
Question 1208449: 2. In the given figure
3. P, Q and R are three points
(a) the bearing of Q from R,
(b) the height of the mast,
(c) the angle of elevation of T from R.
4. A man on a ship
5. A, B, C and D are four points
(a) the bearing of C from B,
(b) the bearing of D from A.
6. A vertical mast
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
One and ONLY ONE problem/question per post.
It is the RULE, the POLICY and the REQUIREMENT of this forum.
It is written in this page
https://www.algebra.com/tutors/students/ask.mpl?action=ask_question&topic=Equations&return_url=http://www.algebra.com/algebra/homework/equations/
from which you post your problems.
It is assumed that you read these rules before posting.
It is also assumed that you do understand what is written in that page and follow the rules.
This rule is established for benefits of visitors (to avoid mess in responses).
Those who violate them, work against their own interests.
Question 1208438: A person throws a ball upward into the air with an initial velocity of 11 m/s. How high will it go before it comes to rest?
Answer by ikleyn(51772)   (Show Source): (Show Source):
Question 1208398:
Answer by ikleyn(51772)   (Show Source): (Show Source):
Question 1208257: draw the graph of
2sin3(theta+pi/4)
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Go to web-site www.desmos.com/calculator
Find there free of charge online plotting tool.
It is free of charge and intended for common use.
Print the formula for your function y = 2*sin(3(x+3.14/4)) there and get the plot instantly.
Question 1208087: If John stands at 6ft and has a view of 30 miles, what is his view if he is elevated to 100ft??
Found 2 solutions by ikleyn, josgarithmetic:
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
If John stands at 6ft and has a view of 30 miles, what is his view if he is elevated to 100ft??
~~~~~~~~~~~~~~~~~~~~~~
In this problem, there is NO logical or physical connection between the given part and the question.
So, there is no any basis/reason to write that proportion, which @josgarithmetic uses in his post.
As given in the post, the problem is ILLOGICAL and therefore DEFECTIVE / (absurdist).
Answer by josgarithmetic(39546)  (Show Source): (Show Source):
Question 1208069: what is the possible values of x in 2sin^2(x-30)=cos 60
Found 2 solutions by greenestamps, ikleyn:
Answer by greenestamps(13008)   (Show Source): (Show Source):
You can put this solution on YOUR website!
For tutor @ikleyn....
Currently your post only shows solutions for sin(x-30) = 1/2; you left out the solutions for sin(x-30) = -1/2
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
what  are the possible values of x in 2sin^2(x-30)=cos 60 are the possible values of x in 2sin^2(x-30)=cos 60
~~~~~~~~~~~~~~~~~~~~~
They want you find all real solutions to this equation
2sin^2(x-30) = cos(60). (1)
We have
cos(60) = 1/2; so, this equation takes the form
2sin^2(x-30) = 1/2.
Simplify it step by step
sin^2(x-30) = 1/4,
sin(x-30) = +/- },
sin(x-30) = +/- 1/2. (2)
From this equation, there are two infinite sets of roots for x-30 to equation (2)
x-30 = },
sin(x-30) = +/- 1/2. (2)
From this equation, there are two infinite sets of roots for x-30 to equation (2)
x-30 =  , k = 0, +/-1, +/-2, . . .
x-30 = , k = 0, +/-1, +/-2, . . .
x-30 =  , k = 0, +/-1, +/-2, . . .
It gives two infinite sets of roots for x to equation (1)
x = , k = 0, +/-1, +/-2, . . .
It gives two infinite sets of roots for x to equation (1)
x =  , k = 0, +/-1, +/-2, . . .
x = , k = 0, +/-1, +/-2, . . .
x =  , k = 0, +/-1, +/-2, . . .
ANSWER. Equation (1) has two infinite sets of solutions
x = , k = 0, +/-1, +/-2, . . .
ANSWER. Equation (1) has two infinite sets of solutions
x =  , k = 0, +/-1, +/-2, . . .
x = , k = 0, +/-1, +/-2, . . .
x =  , k = 0, +/-1, +/-2, . . . , k = 0, +/-1, +/-2, . . .
Solved.
======================
To tutor @greenestamps and to all other readers of this post.
Thank you for your attention, but my solution, as it is/was written by me
from the very beginning, is correct and includes all the cases sin(x-30) = +/- 1/2.
It is because the general formula uses not full period  , but half period , but half period  , which absorbs/covers all these cases. , which absorbs/covers all these cases.
So, everything is and was correct in my post. There is no reason to worry.
This alarm from @greenestamps is false.
Question 1207913: Use the information above and the trigonometric identities to prove that A sin(wt+ ∅)= csub2 sinwt+ csub1 wt.
Answer by ikleyn(51772)   (Show Source): (Show Source):
You can put this solution on YOUR website! .
Use the information above and the trigonometric identities to prove
that A sin(wt+ ∅)= csub2 sinwt+ csub1 wt.
~~~~~~~~~~~~~~~~~~~~~~~~~~
This formula in the post is written incorrectly.
As written, it can not be proved, since it is incorrect.
Also, in this post there is NO any "information above" to use,
so the informational value of this instruction for a receiver is zero.
Question 1207688: An aeroplane flies 400km from a point O on a bearing of 025 degrees to a point X,and it then flies 700km on a bearing of 080 degrees to arrive at y
(1) Using a scale of 1cm to represent 100km,draw accurately the positions of the points
(2)Find the distance of y from O
(3)Find the bearing of y from O
Answer by mananth(16944)   (Show Source): (Show Source):
Question 1169794: The angles of elevation of the top 𝘏 of a vertical pole HO are observed to be θ and α from points 𝘗 and 𝘘 due east and due south of the post. If the distance PQ = 𝘥, show that the height of the post is
𝘥/√(cot²α + cot²θ)
Answer by mananth(16944)   (Show Source): (Show Source):
Question 1207667: Chidi measured the angle of elevation of a tree and found it was 38 degrees. He then walked 25m towards the tree and measured the angle of elevation again to be 50 degrees (1)Sketch the diagram (2)use a scale of 1cm to represent 10m to draw the diagram accurately. (3)Find the actual height of the tree (4)find the total distance to the bottom of tree from chidi's initial position
Answer by josgarithmetic(39546)  (Show Source): (Show Source):
Question 1207649: prove that (\sin (\theta )+\tan (\theta ))/(\csc (\theta )+\cot (\theta )) = sin(\theta ) tan (\theta )
Found 2 solutions by Edwin McCravy, MathLover1:
Answer by Edwin McCravy(19937)   (Show Source): (Show Source):
Answer by MathLover1(20819)   (Show Source): (Show Source):
Question 1207608: Two sides and an angle are given below. Determine whether the given information results in one triangle, two triangles, or no triangle at all. Solve any resulting triangle(s).
b=8, c=9, B=40degrees
Answer by MathLover1(20819)   (Show Source): (Show Source):
Question 1207609: Two sides and an angle are given below. Determine whether the given information results in one triangle, two triangles, or no triangle at all. Solve any resulting triangle(s).
a=1, b=5, A=60degrees
Answer by MathLover1(20819)   (Show Source): (Show Source):
Question 1207443: Given that θ is acute and tan𝜃=12/5, find the values of
sinθ, cos𝜃, csc𝜃, sec𝜃, and cot𝜃.
Found 2 solutions by Edwin McCravy, MathTherapy:
Answer by Edwin McCravy(19937)   (Show Source): (Show Source):
Answer by MathTherapy(10531)   (Show Source): (Show Source):
|
Older solutions: 1..45, 46..90, 91..135, 136..180, 181..225, 226..270, 271..315, 316..360, 361..405, 406..450, 451..495, 496..540, 541..585, 586..630, 631..675, 676..720, 721..765, 766..810, 811..855, 856..900, 901..945, 946..990, 991..1035, 1036..1080, 1081..1125, 1126..1170, 1171..1215, 1216..1260, 1261..1305, 1306..1350, 1351..1395, 1396..1440, 1441..1485, 1486..1530, 1531..1575, 1576..1620, 1621..1665, 1666..1710, 1711..1755, 1756..1800, 1801..1845, 1846..1890, 1891..1935, 1936..1980, 1981..2025, 2026..2070, 2071..2115, 2116..2160, 2161..2205, 2206..2250, 2251..2295, 2296..2340, 2341..2385, 2386..2430, 2431..2475, 2476..2520, 2521..2565, 2566..2610, 2611..2655, 2656..2700, 2701..2745, 2746..2790, 2791..2835, 2836..2880, 2881..2925, 2926..2970, 2971..3015, 3016..3060, 3061..3105, 3106..3150, 3151..3195, 3196..3240, 3241..3285, 3286..3330, 3331..3375, 3376..3420, 3421..3465, 3466..3510, 3511..3555, 3556..3600, 3601..3645, 3646..3690, 3691..3735, 3736..3780, 3781..3825, 3826..3870, 3871..3915, 3916..3960, 3961..4005, 4006..4050, 4051..4095, 4096..4140, 4141..4185, 4186..4230, 4231..4275, 4276..4320, 4321..4365, 4366..4410, 4411..4455, 4456..4500, 4501..4545, 4546..4590, 4591..4635, 4636..4680, 4681..4725, 4726..4770, 4771..4815, 4816..4860, 4861..4905, 4906..4950, 4951..4995, 4996..5040, 5041..5085, 5086..5130, 5131..5175, 5176..5220, 5221..5265, 5266..5310, 5311..5355, 5356..5400, 5401..5445, 5446..5490, 5491..5535, 5536..5580, 5581..5625, 5626..5670, 5671..5715, 5716..5760, 5761..5805, 5806..5850, 5851..5895, 5896..5940, 5941..5985, 5986..6030, 6031..6075, 6076..6120, 6121..6165, 6166..6210, 6211..6255, 6256..6300, 6301..6345, 6346..6390, 6391..6435, 6436..6480, 6481..6525, 6526..6570, 6571..6615, 6616..6660, 6661..6705, 6706..6750, 6751..6795, 6796..6840, 6841..6885, 6886..6930, 6931..6975, 6976..7020, 7021..7065, 7066..7110, 7111..7155, 7156..7200, 7201..7245, 7246..7290, 7291..7335, 7336..7380, 7381..7425, 7426..7470, 7471..7515, 7516..7560, 7561..7605, 7606..7650, 7651..7695, 7696..7740, 7741..7785, 7786..7830, 7831..7875, 7876..7920, 7921..7965, 7966..8010, 8011..8055, 8056..8100, 8101..8145, 8146..8190, 8191..8235, 8236..8280, 8281..8325, 8326..8370, 8371..8415, 8416..8460, 8461..8505, 8506..8550, 8551..8595, 8596..8640, 8641..8685, 8686..8730, 8731..8775, 8776..8820, 8821..8865, 8866..8910, 8911..8955, 8956..9000, 9001..9045, 9046..9090, 9091..9135, 9136..9180, 9181..9225, 9226..9270, 9271..9315, 9316..9360, 9361..9405, 9406..9450, 9451..9495, 9496..9540, 9541..9585, 9586..9630, 9631..9675, 9676..9720, 9721..9765, 9766..9810, 9811..9855, 9856..9900, 9901..9945, 9946..9990, 9991..10035, 10036..10080, 10081..10125, 10126..10170, 10171..10215, 10216..10260, 10261..10305, 10306..10350, 10351..10395, 10396..10440, 10441..10485, 10486..10530, 10531..10575, 10576..10620, 10621..10665, 10666..10710, 10711..10755, 10756..10800, 10801..10845, 10846..10890, 10891..10935, 10936..10980, 10981..11025, 11026..11070, 11071..11115, 11116..11160, 11161..11205, 11206..11250, 11251..11295, 11296..11340, 11341..11385, 11386..11430, 11431..11475, 11476..11520, 11521..11565, 11566..11610, 11611..11655, 11656..11700, 11701..11745, 11746..11790, 11791..11835, 11836..11880, 11881..11925, 11926..11970, 11971..12015, 12016..12060, 12061..12105, 12106..12150, 12151..12195, 12196..12240, 12241..12285, 12286..12330, 12331..12375, 12376..12420, 12421..12465, 12466..12510, 12511..12555, 12556..12600, 12601..12645, 12646..12690, 12691..12735, 12736..12780, 12781..12825, 12826..12870, 12871..12915, 12916..12960, 12961..13005, 13006..13050, 13051..13095, 13096..13140, 13141..13185, 13186..13230, 13231..13275, 13276..13320, 13321..13365, 13366..13410, 13411..13455, 13456..13500, 13501..13545, 13546..13590, 13591..13635, 13636..13680, 13681..13725, 13726..13770, 13771..13815, 13816..13860, 13861..13905, 13906..13950, 13951..13995, 13996..14040, 14041..14085, 14086..14130, 14131..14175, 14176..14220, 14221..14265, 14266..14310, 14311..14355, 14356..14400, 14401..14445, 14446..14490, 14491..14535, 14536..14580, 14581..14625, 14626..14670, 14671..14715, 14716..14760, 14761..14805, 14806..14850, 14851..14895, 14896..14940, 14941..14985, 14986..15030, 15031..15075, 15076..15120, 15121..15165, 15166..15210, 15211..15255, 15256..15300, 15301..15345, 15346..15390, 15391..15435, 15436..15480, 15481..15525, 15526..15570, 15571..15615, 15616..15660, 15661..15705, 15706..15750, 15751..15795, 15796..15840, 15841..15885, 15886..15930, 15931..15975, 15976..16020, 16021..16065, 16066..16110, 16111..16155, 16156..16200, 16201..16245, 16246..16290, 16291..16335, 16336..16380, 16381..16425, 16426..16470, 16471..16515, 16516..16560, 16561..16605, 16606..16650, 16651..16695, 16696..16740, 16741..16785, 16786..16830, 16831..16875, 16876..16920, 16921..16965, 16966..17010, 17011..17055, 17056..17100, 17101..17145, 17146..17190, 17191..17235, 17236..17280, 17281..17325, 17326..17370, 17371..17415, 17416..17460, 17461..17505, 17506..17550, 17551..17595, 17596..17640, 17641..17685, 17686..17730, 17731..17775, 17776..17820, 17821..17865, 17866..17910, 17911..17955, 17956..18000, 18001..18045, 18046..18090, 18091..18135, 18136..18180, 18181..18225, 18226..18270, 18271..18315, 18316..18360, 18361..18405, 18406..18450, 18451..18495, 18496..18540, 18541..18585, 18586..18630, 18631..18675, 18676..18720, 18721..18765, 18766..18810, 18811..18855, 18856..18900, 18901..18945, 18946..18990, 18991..19035, 19036..19080, 19081..19125, 19126..19170, 19171..19215, 19216..19260, 19261..19305, 19306..19350, 19351..19395, 19396..19440, 19441..19485, 19486..19530, 19531..19575, 19576..19620, 19621..19665, 19666..19710, 19711..19755, 19756..19800, 19801..19845, 19846..19890, 19891..19935, 19936..19980, 19981..20025, 20026..20070, 20071..20115, 20116..20160, 20161..20205, 20206..20250, 20251..20295, 20296..20340, 20341..20385, 20386..20430, 20431..20475, 20476..20520, 20521..20565, 20566..20610, 20611..20655, 20656..20700, 20701..20745, 20746..20790, 20791..20835, 20836..20880, 20881..20925, 20926..20970, 20971..21015, 21016..21060, 21061..21105, 21106..21150, 21151..21195, 21196..21240, 21241..21285, 21286..21330, 21331..21375, 21376..21420, 21421..21465, 21466..21510, 21511..21555, 21556..21600, 21601..21645, 21646..21690, 21691..21735, 21736..21780, 21781..21825, 21826..21870, 21871..21915, 21916..21960, 21961..22005, 22006..22050, 22051..22095, 22096..22140, 22141..22185, 22186..22230, 22231..22275, 22276..22320, 22321..22365, 22366..22410, 22411..22455, 22456..22500, 22501..22545, 22546..22590, 22591..22635, 22636..22680, 22681..22725, 22726..22770, 22771..22815, 22816..22860, 22861..22905, 22906..22950, 22951..22995, 22996..23040, 23041..23085, 23086..23130, 23131..23175, 23176..23220, 23221..23265, 23266..23310, 23311..23355, 23356..23400, 23401..23445, 23446..23490, 23491..23535, 23536..23580, 23581..23625, 23626..23670, 23671..23715, 23716..23760, 23761..23805, 23806..23850, 23851..23895, 23896..23940, 23941..23985, 23986..24030, 24031..24075, 24076..24120, 24121..24165, 24166..24210, 24211..24255, 24256..24300, 24301..24345, 24346..24390, 24391..24435, 24436..24480, 24481..24525, 24526..24570, 24571..24615, 24616..24660, 24661..24705, 24706..24750, 24751..24795, 24796..24840, 24841..24885, 24886..24930, 24931..24975, 24976..25020, 25021..25065, 25066..25110, 25111..25155, 25156..25200, 25201..25245, 25246..25290, 25291..25335
|







 .
. .
.