Heisenberg Uncertainty principle defined as $\Delta x \Delta p_x \geq h$ versus $\Delta x \Delta p_x \geq \hbar/2$ [duplicate]

Question

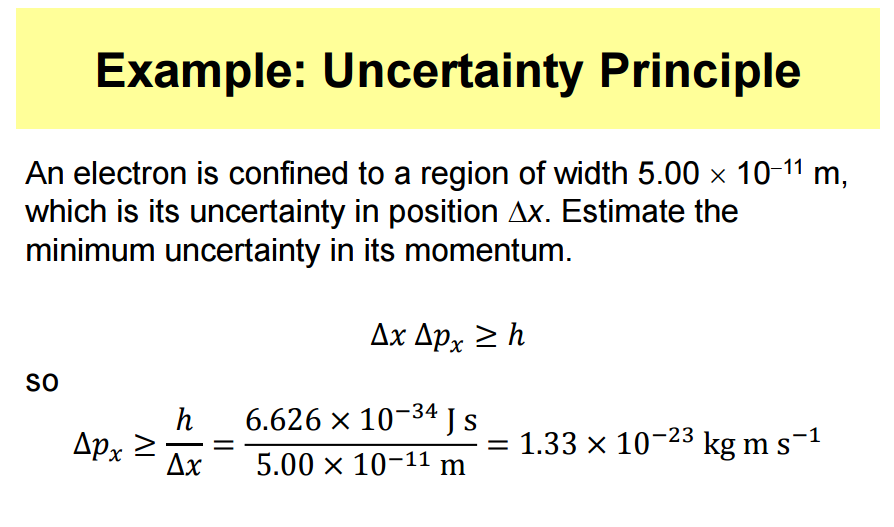
At my university, in the during lectures and in the equation sheet for our exams, the formula for the Heisenberg Uncertainty Principle is stated as $\Delta x \Delta p_x \geq h$, for example in one of my lecture notes, the following example is illustrated using this formula

However I know that in my textbook (University Physics by Young and Freedman) and pretty much universally the Heisenberg Uncertainty Principle is stated as $\Delta x \Delta p_x \geq \frac{\hbar}{2}$. Using this formula, we can see the example above is off by a factor of $4\pi$.

Is the formula, $\Delta x \Delta p_x \geq h$, that my university uses a valid formula? If so is just a weaker version of $\Delta x \Delta p_x \geq \frac{\hbar}{2}$?

Answer 1

The Heisenberg uncertainty principle (HUP)is encoded formally in the theory of quantum mechanics in the commutations relations of the operators defining the observables. There are a number of other observable variable pairs whose commutator is not zero

Commutators use $\hbar$ so it is the "correct" form in the calculations. On the other hand the HUP, when it was first proposed, was an extension of wave mechanics arguments and there a factor of $\pi$ or so was irrelevant. In the formal mathematics, one should use $\hbar$. See also the answer here.