Preprint
Article
Tolman VI fluid sphere in f(R,T) gravity
Altmetrics
Downloads
150
Views
27
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
20 January 2023
Posted:
23 January 2023
You are already at the latest version
Alerts
Abstract
We analyze the behavior of relativistic spherical objects within the context of modified $f(R,T)$ gravity considering Tolman VI spacetime, where gravitational lagrangian is a function of Ricci scalar(R) and trace of energy momentum tensor(T) i.e,$ f(R,T)= R+ 2\beta T$, for some arbitrary constant $\beta$. For developing our model, we have chosen $\pounds_{m} = -p$, where $ \pounds_{m}$ represents matter lagrangian. For this investigation, we have chosen three compact stars namely PSR J1614-2230 [Mass=(1.97$\pm$ 0.4)M$_\odot$; Radius= 9.69$_{-0.02} ^{+0.02}$ Km] ,Vela X-1 [Mass=(1.77$\pm$ 0.08)M$_\odot$; Radius= 9.560$_{-0.08} ^{+0.08}$ Km] and 4U 1538-52 [Mass=(9.69)M$_\odot$; Radius= 1.97 Km]. In this theory the equation of pressure isotropy is identical to standard Einstein's theory. So all known metric potential solving Einstein's equations are also valid here. In this paper, we have investigated the effort of coupling parameter ($\beta$) on the local matter distribution. Sound of speed and adiabatic index are higher with grater values of $\beta$ while on contrary mass function and gravitational redshift are lower with higher values of $\beta$ . For supporting the theoretical results, graphical representation are also employed to analyze the physical viability of the compact stars.
Keywords:
Subject: Physical Sciences - Astronomy and Astrophysics
1. Introduction
The analysis of the interior of the stars is fascinating to astrophysicists, mainly to the General theory of relativity (GR). For the fact that, about the late phase of stellar evolution, general relativistic effects much more important. In this direction, one of the incredible works was that of the Tolman [1] solution (1939). Tolman extensively deliberated the stellar interior and gave us a explicit solutions for the static, spherically symmetric equilibrium fluid distribution [2]. In different dimension, it has been tested in which include cosmology, gravitational waves, astrophysics and thermodynamics [3] of the stellar system and it has present important contributions to the different astrophysics and cosmological issues. Many of them present collapsing of wormhole solution with static spherically symmetric geometry [4] and non static spherically symmetric object with anisotropic fluid profile. Moraes and his co-authors [5] studied modified Tolman-Oppenheimer-Volkoff (TOV) equation in which they illustrates the equilibrium conditions of the compact structures.
In the modifying form of gravitational action asks for lots of fundamental challenges. These models can show ghost-like behavior, instabilities, while on the other side, it has to match with experiments and observations in the low energy limit. Also in the framework of gravity some interesting results have been found at solar system [6], galactic and cosmological scales.
Several models exist which attempt to explain the early acceleration of the universe. The most accepted models contain a slowly varying potential and a scalar field. There are another class of models where the gravity is modified under the general relativity. One of the procedure of the modifications depended upon phenomenological considerations is provided by theory of gravity. Indeed, theories are conformally identical to Einstein’s theory plus a scalar degree of freedom classified the scalar in which potential is uniquely established from Ricci scalar. There are various model, in the literature, where the authors [7] considered Einstein equation with corrections. The consistent theory of gravity, modified or classified, should be equally suitable to the strong gravity regime.
Here is a analytic (general) function of R (Ricci scalar). As an example, cosmological solutions gives the accelerated expansion of the universe at late times. Also it have been found that many stability conditions may lead to avoid tachyon and ghost solution. In addition, there exist viable models satisfying both stability conditions [8] and background cosmological constraints and results have been obtained to place constants on cosmological model by cosmic microwave background radiation (CMBR) galaxy and anisotropic power spectrum [9,10]. To consider gravity in low energy limit, it is viable to obtain accurate gravitational potentials capable of describing the flat rotational curves of the dynamics of galaxy or spiral galaxies clusters without considering large amount of dark matter [11].
Numerous investigations [12,13,14,15,16] have used different method to examined the stability as well as consistency of gravity theory. There are definite form of algebraic function which eliminated the existence of stable astrophysical form and reported unrealistic. In recent years, more research has been performed on the steadiness, dynamical unsteadiness, existence of celestial stellar system of this theory [17,18,19]. Harko et.all [20] proposed the concept of matter and curvature couplings to represent a new version of altered theory of gravity, namely gravity. They also represented the relating field equation with the help of gravitational potential mechanism and showed the important of alternative gravity theory. Also the same authors have initiated various model for algebraic functional for detachable compose viz.= +. Houndjo [21] investigated matter instructed age of accelerating cosmic by gravity. Also, Baffou and his teammates [22] examined spatially uniform cosmic in the field of gravity.
Modified and extended models are always popular due to the potential of representation of gravitational field nature near curvature singularities accurately and as well to overcome the cosmological constant problems. Convincing confirmation for the extension of the universe has been provided by the many independent observations, some of these are supernovas Ia data [23], cosmic microwave background radiation [24] and baryon acoustic oscillation [25] according to the study by the WMAP. For addressing this phenomenon, several assumption have been suggested from dark energy model to modified theories of gravity . Nowadays, dark energy model have no sufficient observational support. In particular, the dark energy idea requires an eqn. of state (EoS) , where represents spatially homogeneous pressure, energy density , respectively and the value of the parameter is . Several results have initiated for interior exact solutions of the Einstein field equation and Schwarzschild found the first interior solution. Tolman proposed an inventive method for treatment of the Einstein field equation which are known as Tolman I, II, III, IV, V, VI, VII and VIII [26].
In this paper, we consider Tolman VI model [27] in the class of modified gravity in which the gravitational action carries a general function . For this model, the study of the background cosmological evolution can be simplified by performing transformation on metric. Such type of transformation maps from a frame where the resulting field equations and gravitational action are modified from general relativity (GR), called the Jordan frame, to a frame where the gravitational action for the new obtain metric is the Einstein-Hilbert one, called the Einstein frame. The gravity theory has been related to stellar astrophysics [28] and cosmology [29], among other areas, giving testable and interesting results.
The present article deals with isotropic Tolman VI in modified gravity. The physical characteristics of our obtained model are studied for three compact stars PSR J1614-2230, Vela X-1 and 4U 1538-52. The paper is organized in following order of sections. Sec. II, we explain about the general formalism of gravity and in section III, the proposed model is obtained for different values of coupling parameter . At the boundary, we matched our interior space-time to the exterior space-time in section IV. Section V explains the physical properties between Einstein theory and gravity. Finally in section VI, we discuss and concludes the whole work by pointing on major findings.
2. Mathematics Behind f(R,T) Gravity
In this section we devote how the f(R,T) was introduced. The Ricci scalar is integrated over a four dimensional volume element when Einstein’s field equation is derived from Einstein-Hilbert action as
If we replace the Ricci scalar R by f (R,T), We can get the f(R,T) field equations. Therefore, the complete action in f (R,T) formalism is
where, T is the trace of the energy momentum tensor . Also, represents the Lagrangian matter dencity and .
The energy momentum tensor is defined as
along with the trace . Also the Lagrangian density depends only the metric tensor component , not its derivatives. Here, we have
By variation principle w.r.t , Equation (2) gives the field equation
where, and Here, covariant derivative is associated with Levi-Civita connection of the metric tensor and the box operation □ is defined as with The covariant derivative of the Equation (5) gives
In gravity, the stress-energy tensor of the matter field do not obey the conservation low due to interaction between the curvature and matter as in general relativity. With the help of the Equation (3), we get the tensor as follows
for the field equation, we assume the energy-momentum tensor as
provided, the four velocity, such that and with and are matter density, radial pressure and transverse pressure, respectively. If we specify pressure as , the Equation (7) reduces to
3. Interior space-time and the realistic viable f(R,T) gravity models:
We will represent the model with the help of realistic f(R, T ) gravity model. Here, we consider a separable functional form of f(R, T ) given by,
in the relativistic structures to study the coupling effects of matter and curvature components in f(R, T ) gravity, where and representing arbitrary functions of R and T, respectively. Several viable model in f(R, T ) gravity can be generated in linear combining of different forms of and . In the present model, we assume and . Then the expression for becomes
where, is arbitrary constant to be evaluated depending on many physical requirements.
In curvature coordinate, we consider the static and spherically symmetric line element describing a wormhole region by the following metric:
where, both depends on r, i.e both are purely radial and . In modified gravity, the field equation along the line element (12) can be written as
where a prime (′) denotes differentiation with respect to the radial coordinates ‘r’.
We denote and by,
where, represents the density and represents the pressure in Einstein gravity. To solve the Equations (13)−(15) we use the metric potential by Tolman [1] in which the expression
where, A,B are arbitrary constant. The restriction of is , but this is not most general choice. Using the expression of the Equation (13) and (18), we obtain the Einstein density as
Similarly, using the expression of the Equations (14), (18) and (19), we obtain the Einstein pressure as follows
If we eliminate radius r from (20) and (21), we obtain the relation between Einstein density and pressure. Also, the positivity of density profile demands the range for n are or or . So the interval of validity is . Now, using the expression and from (16) and (21), we get the expression for matter density and pressure in modified gravity as follows
where,
The square of the sound velocity for Einstein and our present model are obtained as follows
Negative sign in the sound speed index can be removed in the interval . The profile of density and pressure are shown in Figure 1 and Figure 2, respectively. One can see that the density and pressure are both positive definite but at the stellar center both are infinite.
In the literature, it is well-known that the mass distributions must obey all the energy conditions in its interiors. These energy conditions are named as null, strong, week and dominant energy conditions and symbolized by NEC, SEC, WEC and DEC. All the energy conditions are satisfied for our present model if the following inequality are hold.
NEC: , SEC: , , WEC: , , DEC:, .
It is clear from Figure 3 that , in the Figure 4, and is non negative shown in the Figure 5. So all the necessary energy conditions have been fulfilled for our gravity model.
Figure 3.
Behavior of the “Speed sound” with respect to the radial coordinate “ r” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 3.
Behavior of the “Speed sound” with respect to the radial coordinate “ r” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .

Figure 4.
Variation of “ adiabatic index” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 4.
Variation of “ adiabatic index” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .

Figure 5.
Behavior of the “Week energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 5.
Behavior of the “Week energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
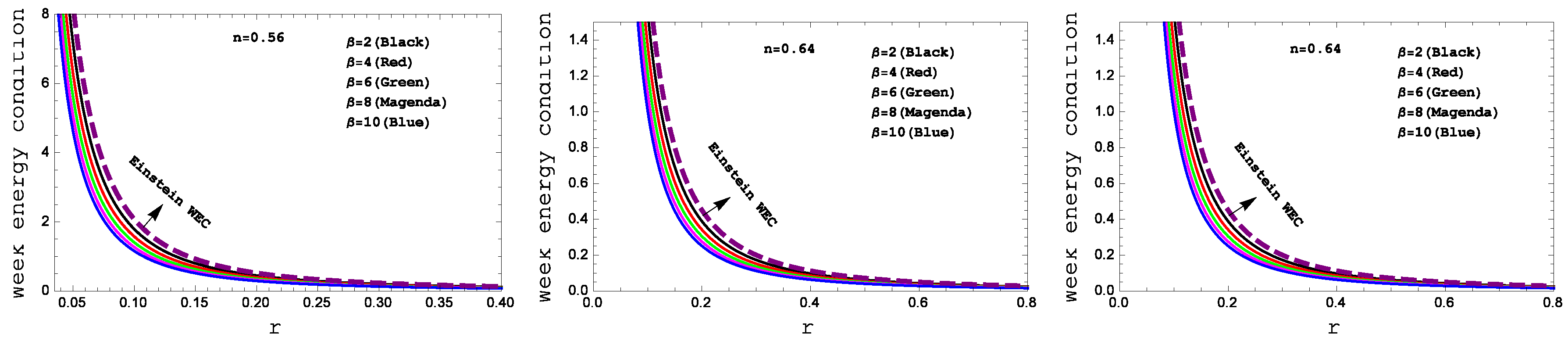
Figure 6.
Behavior of the “Strong energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 6.
Behavior of the “Strong energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .

Figure 7.
Behavior of the “Dominant energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 7.
Behavior of the “Dominant energy condition” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .

4. Exterior space-time and boundary condition:
Now, we have matched our interior space-time to exterior Schwarzschild line element at the .
The Exterior line element is
and the interior line element is
The continuity of the metric
The pressure vanishes at the boundary i.e which gives the following equation in modified gravity as follows
- Determination of n and the constants A and B: Solving the Equations (36)–(38), we get the expression for n and the constants A and B as follows:
5. Physical properties of the present model:
-
Nature of equation of state: It is very important to describe a relationship between energy density and the pressure which is called the equation of state(EoS). The relation between the pressure and matter density can be find out by dimensionless quantity which is known as the equation of state parameter.Hence, the equation of state parameter(w) for Einstein and our model are obtained as followsThe behavior of equation of state parameter is shown in the Figure 8. We can see that equation of state parameter is monotonic decreasing function of radius r.Figure 8. Behavior of the “EoS parameter” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .Figure 8. Behavior of the “EoS parameter” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .

- Relativistic adiabatic index: For a compact star, stability is one of the most crucial requirement. For this reason we have discussed stability along with the variation of adiabatic index inside the compact star. The adiabatic index can be displays the stability for both non-relativistic and relativistic compact stars. The stability condition for a Newtonian sphere is and is the condition for a neutral equilibrium according to [30]. The expression relativistic adiabatic index for Einstein and our present model are
-
TOV Equation: The hydrostatic equilibrium equation is an important feature of the physical realistic compact objects. The fluid sphere remains at equilibrium under three forces namely, gravitational force, hydrostatic force and the additional force due to modified gravity and this situation represents by an equation, which is known as Tolman-Oppenheimer-Volkov (TOV) equation. With the help of generalized TOV equation, we can analyze the equilibrium equation for our three compact stars. The generalized TOV eqn. for the isotropic fluid [31] distribution in f(R, T ) modified gravity can be written asSince, corresponds to GR. Hence , the TOV Equation for Einstein reduces to
- Mass radius relationship and compactness parameter : let be the compactification factor and M be the mass function. Then we can get following relation between them,where, and represents the hypergeometric function. The expression for mass function for Einstein and our present model are
-
Gravitational red-shift() function and surface red-shift() : The gravitational redshift can be determined by the formulaFurthermore, the following formula can be used to calculate surface redshift for Einstein and our present model are
6. Discussion and concluding remarks
In this present work, we have investigated the behavior of Tolman VI spacetime in modified gravity. We endeavored to solve the modified field equations and investigated the physical viability according to the standard theory. We contrasted the behavior of the matter energy density, isotropic pressure, the sound speed energy, the all energy conditions (namely, weak, strong and dominated energy condition), EoS parameter, mass profile as well as gravitational redshift between the modified theory and standard Einstein theory.
For the arbitrary constant and 10 the graphical picture have been presented in the Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9 and Figure 10 for the compact stars , and while, gives the General relativity case.
Figure 9.
Behavior of the “mass profile” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 9.
Behavior of the “mass profile” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel)corresponding to the numerical value of constants A and B from the table II and for different values of .

Figure 10.
Behavior of the “Gravitational red-shift” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 10.
Behavior of the “Gravitational red-shift” with respect to the radial coordinate “ r ” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .

- A clear picture of energy progression has been obtained in Figure 1. The figure shows declining nature about the surface and promises the real origination of stellar body with positive behavior at the stellar interior.
- We have plotted pressure p versus radius r in Figure 2 for the three compact stars PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) for various values of . One can see that i.e positive, continuous and monotonically decreasing. Also, at some radial value, the pressure does vanish for both the cases.
- Square of the sound speed and relativistic adiabatic index have been plotted in Figure 3 and Figure 4, respectively. From the Figure 3, one can see that the square of the sound speed lies in the predicted range i.e. throughout the fluid sphere. The Figure 4 confirms the stability of under the adiabatic index for our present model.
- In our gravity model, the weak energy condition (WEC) in Figure 5, the strong energy condition (SEC) in Figure 6 and dominant energy condition (DEC) in Figure 7 are also met. For the complication in the expressions of density and pressure we have shown graphical presentation which certifies about the well behaved nature of the energy conditions.
- We have plotted equation of state parameter profile in Figure 8 for different values of . It is clear from the figure that at the center of the star these parameter take maximum values while it decreases towards the boundary. Moreover, lies between 0 to 1 i.e which indicates the non-exotic behavior of matter distribution. Also, we can see that there are linear relation between isotropic pressure (p) and matter density ().
- The mass function is plotted against radius in Figure 9. This figure shows that mass function is monotonic increasing function of radius and having no central singularity. The mass functional values are in agreement with required physical conditions as one can investigate from the figure.
- We have plotted the Gravitational redshift in Figure 10 for different values of . One can see that Gravitational redshift is monotonic decreasing function of radius. Also, gravitational redshift is lower with higher values of coupling parameter
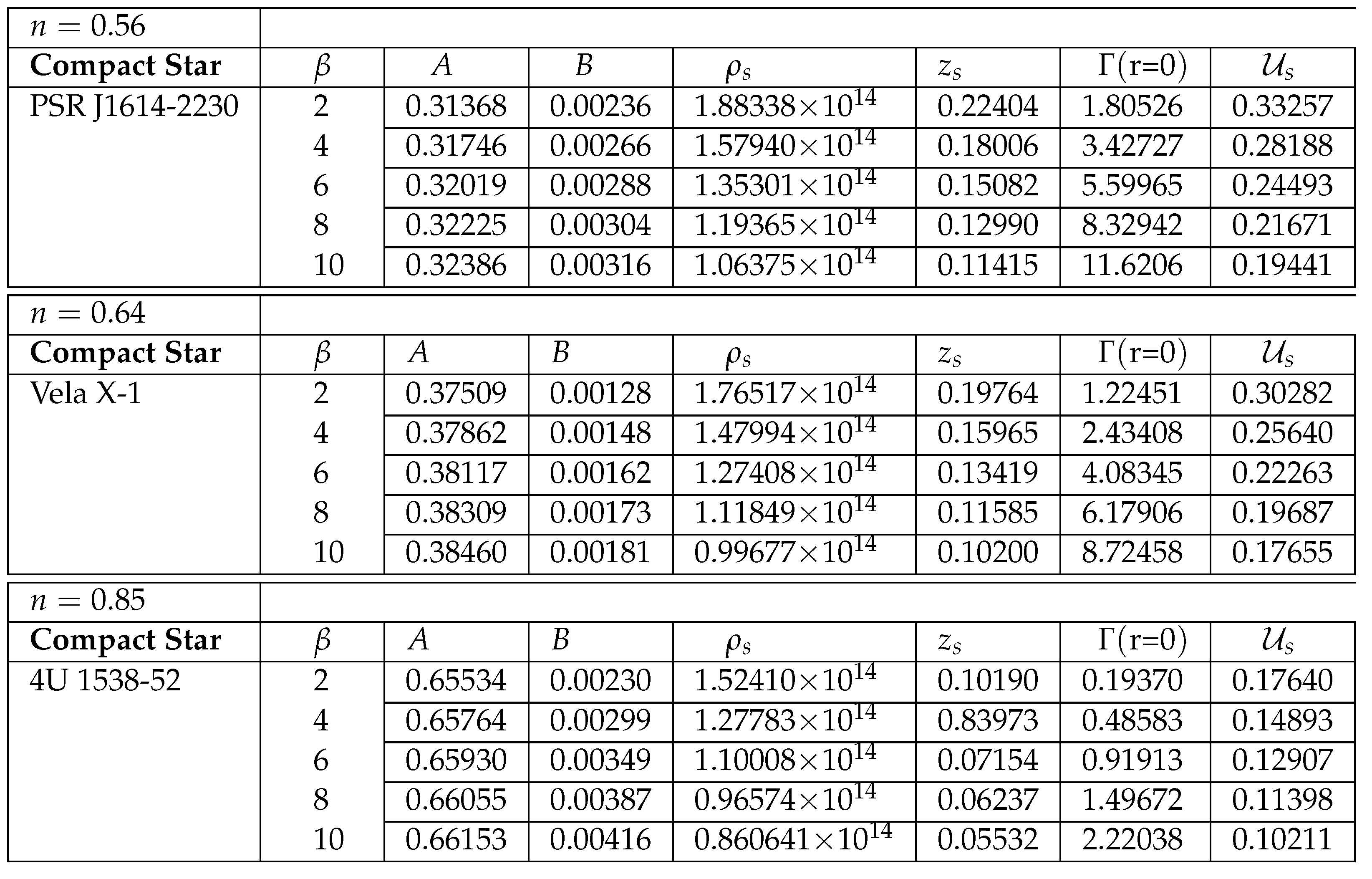
From all graphical illustrations and obtained results, we can conclude that our present model is regular and potentially stable. Also, detailed numerical features can be found from Table 1 and Table 2. The numerical values of A and B increases with increasing values of . The surface density , and surface red-shift all takes lower values when coupling parameter increases. Moreover, the central values of adiabatic index ( at ) increase with increasing values of , which concludes that for higher values of our model becomes more stable. Through analytical, numerical and graphical analysis, all the features of our present model are well described. Finally, we summarize our discussion that we are convinced by the calculated outcomes which shows that the system is physically reasonable and viably stable. Also, our outcomes could be useable in modeling relativistic compact objects as a real astrophysical phenomena.
Table 1.
Numerical values of constants for three well-known celestial compact stars.

Table 2.
Numerical values of constants for three well-known celestial compact stars.
Acknowledgments
FR would like to thank the authorities of the Inter-University Centre for Astronomy and Astrophysics, Pune, India for providing research facilities.
Data Availability Statement
No Data associated in the manuscript.
References
- R.C. Tolman, Phys. Rev., 55, 364 (1939).
- S. Ray and B. Das, Mon. Not. R. Astron. Soc. 349, 1331–1334 (2004).
- M. E. S. Alves, P. H. R. S. Moraes, J. C. N. de Araujo, M. Malheiro, Phys. Rev. D, 94, 024032(2016).
- P. H. R. S. Moraes and P. K. Sahoo, Phys. Rev. D, 97, 024007 (2018).
- P.H.R.S. Moraes, J.D.V. Arbanil, M. Malheiro, J. Cosmol. Astropart. Phys., 06 , 005(2016).
- T. Manna, F.Rahaman, M. Mondal, Modern Physics Letter A, 2050034(2019).
- P. Rej, P. Bhar; arXiv:2105.12572v2 [gr-qc] 1 Jun 2021.
- B.Li, Barrow, J.D.: Phys. Rev. D, 75, 084010 (2007).
- R.Bean, D. Bernat, L. Pogosian, A. Silvestri, M. Trodden, Phys. Rev. D, 75, 064020 (2007).
- M. Mondal, F. Rahaman, K.N Singh, Eur. Phys.J.C, 81, 84 (2021).
- S. Das, N. Sarkar, M. Mondal and F. Rahaman, Mod. Phys. Lett. A, 35, 2050280 (2020).
- A.de la Cruz-Dombriz and A. Dobado, Phys. Rev. D, 74, 087501(2006).
- S.E. Perez Bergliaffa, Phys. Lett. B, 642, 311 (2006).
- M. Mondal, P. Pradhan, F. Rahamann, I. Karar, Mod. Phys. Lett. A, 2050249 (2020).
- F. Rahamana, T. Manna, R. Shaikh, S. Aktar, M. Mondal, B. Samanta, Nuclear Physics B, 972, 115548 (2021.).
- M. Mondal, A. K.Yadav, P. Pradhan, S. Islam and F. Rahaman, International Journal of Modern Physics D, Vol 30, No. 12, 2150095 (2021).
- A. Ganguly, R. Gannouji, R. Goswami and S. Ray, Phys. Rev. D, 89, 064019 (2014).
- R. Goswami, A.M. Nzioki, S.D. Maharaj and S.G. Ghosh, Phys. Rev.D, 90, 084011 (2014).
- A. K. Yadav, M. Mondal and F. Rahaman, Pramana J. Phys., 94, 90(2020).
- T. Harko, et al., Phys. Rev. D, 84, 024020 (2011).
- M. Houndjo Int. J. Mod. Phys. D 21, 1250003 (2012).
- E. H. Baffou, A. V. Kpadonou, M. E. Rodrigues, M. J. S. Houndjo, and J. Tossa Astrophys. Space Sci., 356, 173 (2015).
- S. Perlmutter et al., Astrophys. J., 517, 565 (1999); A.G. Riess et al., Astron. J., 116, 1009 (1998); J. L. Tonry et al., Astrophys. J., 594, 1 (2003); R. A. Knop et al., Astrophys. J., 598, 102 (2003); A. G. Riess et al., Astrophys. J., 607, 665 (2004); G. Hinshaw et al., Astrophys. J. Suppl. Ser., 148, 135 (2003).
- D. N. Spergel et al., Astrophys. J. Suppl. Ser., 148, 175 (2003); E. Komatsu et al., Astrophys. J. Suppl. Ser., 192, 18(2011).
- D. J. Eisenstein et al. (SDSS Collaboration), Astrophys. J., 633, 560 (2005); W. J. Percival et al., Mon. Not. R. Astron. Soc., 401, 2148 (2010).
- S. Hansraj and A. Banerjee, physical review D, 97, 104020 (2018).
- S. Ray and B. Das; arXiv:astro-ph/0409527v2 31 Oct 2007.
- D. Deb et al., Phys. Rev. D, 97, 084026(2018).
- K.N. Singha, S. K. Mauryab, A. Errehymyc, F.Rahamand and M. Daoude, Physics of the Dark Universe, Vol 30, 10062( 2020).
- H. Bondi Prac. R. Soc. Lond. A , 281, 39 (1964).
- P. Bhar et al., arXiv:2112.07581v1[gr-qc] 14 Dec 2021.
Figure 1.
Behavior of the “energy density” with respect to the radial coordinate “” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 1.
Behavior of the “energy density” with respect to the radial coordinate “” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .

Figure 2.
Behavior of the “pressure” with respect to the radial coordinate “” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .
Figure 2.
Behavior of the “pressure” with respect to the radial coordinate “” for the compact star PSR J1614-2230(left panel), Vela X-1(middle panel) and 4U 1538-52(right panel) corresponding to the numerical value of constants A and B from the table II and for different values of .

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Tolman VI fluid sphere in f(R,T) gravity
Monimala Mondal
et al.
,
2023
Study on Anisotropic Strange Stars in f(T,T) Gravity
Ines G. Salako
et al.
,
2020
Trajectory of Massive Particles around a Static Black Hole in f(R) Gravity
Surajit Mandal
,
2022
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated








