Fourier series

GUJARAT POWER ENGINEERING
AND
RESEARCH INSTITUTE
ADVANCED
ENGINEERING
MATHEMATICS
FOURIER SERIES

GROUP MEMBERS
 Pinky Chaudhari (131040109006)
 Harwinder Kaur(131040109015)
 Vibha Patel (131040109044)
 Samia Zehra (131040109052)
 Guided By : Prof. Nirav S. Modi

INDEX
 Fourier Series
 General Fourier
 Discontinuous Functions
 Change Of Interval Method
 Even And Odd Functions
 Half Range Fourier Cosine & Sine Series

FOURIER SERIES
 A Fourier series is an expansion of a periodic
function in terms of an infinite sum
of sines and cosines.

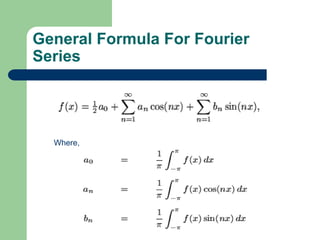
General Formula For Fourier
Series
Where,

Formulas To Solve Examples
 2SC = S + S
 2CS = S – S
 2CC = C + C
 2SS = cos(α-β) –cos(α+β)
 Even*Odd = Odd
 Even*Even = Even
 Odd*Odd = Even
 Odd*Even = Odd

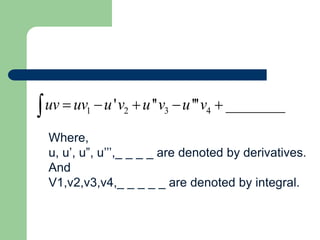
uv  uv1 u 'v2 u''v3 u'''v4  ________
Where,
u, u’, u”, u’’’,_ _ _ _ are denoted by derivatives.
And
V1,v2,v3,v4,_ _ _ _ _ are denoted by integral.

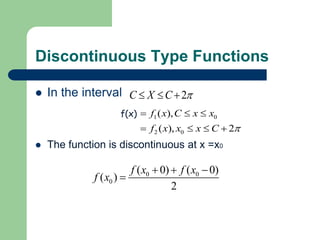
Discontinuous Type Functions
 In the interval
C  X C2
f x C x x
f x x x C 
  
   
( ),
( ), 2
1 0
2 0
f(x)
 The function is discontinuous at x =x0
f x f x
0 0
0
( 0) ( 0)
( )
2
f x
  


So Fourier series formula is
x C
0
2

a f x dx f x dx
 
0 1 2
0
1
( ) ( )
C x

    
   
 
x C
0
2

a f x nx dx f x nx dx
 
1 2
0
x C
0
2

b f x nx dx f x nx dx
 
1 2
0
1
( )*sin( ) ( )*sin( )
n
C x

    
   
 
1
( )*cos( ) ( )*cos( )
n
C x

    
   
 

Change Of Interval Method
 In this method , function has period P=2L ,
where L is any integer number.
 In interval 0<x<2L Then l = L/2
 When interval starts from 0 then l = L/2
 In the interval –L < X < L Then l = L
 For discontinuous function , Take l = C where
C is constant.

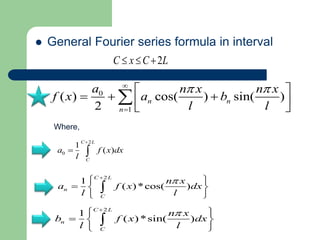
 General Fourier series formula in interval
C  x C 2L
  
a n x n x
f x a b
( ) cos( ) sin( )
2 n n
a f x dx
C L
2
2 1
   
  

2 1
( )*cos( )
   
  ( )*sin( )

C L
n

C
n x
b f x dx
l l
 
n
C
n x
a f x dx
l l
 
0
1
( )
C L
C
l

 
0
1
n
l l

 
    
 

Where,

Even Function
f (x)  f (x)
 The graph of even function is symmetrical
about Y – axis.
 Examples :
2 2 x , x ,cos x, x cos x, xsin x

Fourier series for even function
1. In the interval   x 
  0

( )  
cos( )
1
0


0
2


0
2
n
( )

2
n
( )*cos( )
n
a
f x a nx
a f x dx
a f x nx dx




 
  
 

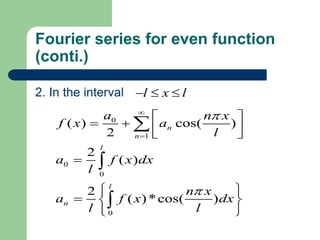
Fourier series for even function
(conti.)
2. In the interval l  x  l
0


 

( ) cos( )
   

1
0

0
2

0
2
n
( )
2
n
 

( )*cos( )
l
l
n
a n x
f x a
l
a f x dx
l
n x
a f x dx
l l

 
  
 

Odd Function
f (x)   f (x)
 The graph of odd function is passing through
origin.
 Examples:-
3 3 x, x , x cos x,sin x, x cos x

Fourier series for odd function
1. In the interval   x 
f x b nx
( ) sin( )
1
b f x nx dx
0
2
n
( ) sin( )
n
n









Fourier series for odd function
(conti.)
 In the interval l  x  l
( ) sin( )
1
0
2
n
( ) sin( )
n
n
n
f x b x
l
n
b f x x dx
l











Half Range Fourier Cosine Series
 In this method , we have 0 < x < π or 0 < x < l
type interval.
 In this method , we find only a0 and an .
 bn = 0

Half Range Fourier Cosine Series
1.In the interval 0 < x < π
  0

( )  
cos( )
1
0


0
2


0
2
n
( )

2
n
( )*cos( )
n
a
f x a nx
a f x dx
a f x nx dx




 
  
 

Half Range Fourier Cosine
Series(conti.)
2. In the interval 0 < x < l
Take l = L
0


 

( ) cos( )
   

1
0

0
2

0
2
n
( )
2
n
 

( )*cos( )
l
l
n
a n x
f x a
l
a f x dx
l
n x
a f x dx
l l

 
  
 

Half Range Fourier Sine Series
 In this method , we find only bn
 an =0
 a0 =0

1. In interval 0 < x < π
f x b nx
( ) sin( )
1
b f x nx dx
0
2
n
( ) sin( )
n
n









(conti.)
2. In the interval 0 < x < l
( ) sin( )
1
0
2
n
( ) sin( )
n
n
n
f x b x
l
n
b f x x dx
l











Fourier series

More Related Content

Fourier series